Hi everyone and welcome to MathSux! In this weeks post, we will venture into Calculus for the first time! I won’t get too much into the nitty gritty explanation of what derivative are here, but instead will provide a nice overview of Derivatives Practice Questions. This post includes everything you need to know about finding the derivatives of a function including the Power Rule, Product Rule, Quotient Rule, and the Chain Rule. Below you will see examples, a Derivative Rules Cheat Sheet, and of course practice questions! I hope these quick examples help in the classroom or for that test coming up! Let me know if it helps and you want more Calculus lessons. Happy Calculating!
What is a Derivative?
We use the derivative to find the rate of change of a function with respect to a variable. You can find out more about what a derivative is and its proper notation here at mathisfun.com. Read on below for a derivative rules cheat sheet, examples, and practice problems!
Derivative Rules Cheat Sheet:
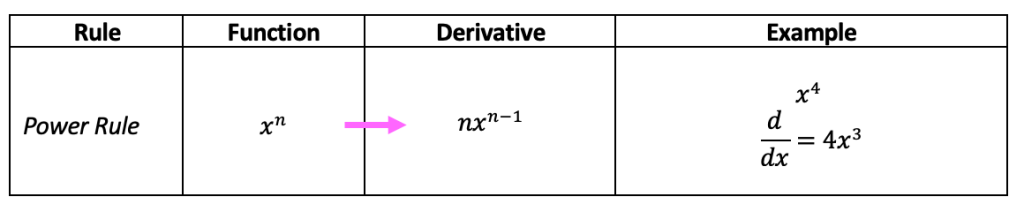
Power Rule:
The power rule is used for finding the derivative of functions that contain variables with real exponents. Note that the derivative of any lone constant number is zero.
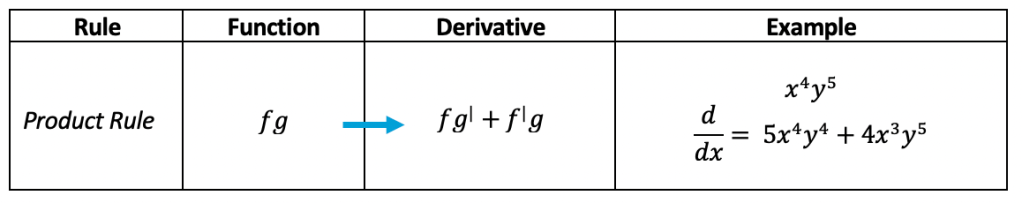
Product Rule:
The product rule is used to find the derivative of two functions that are being multiplied together.
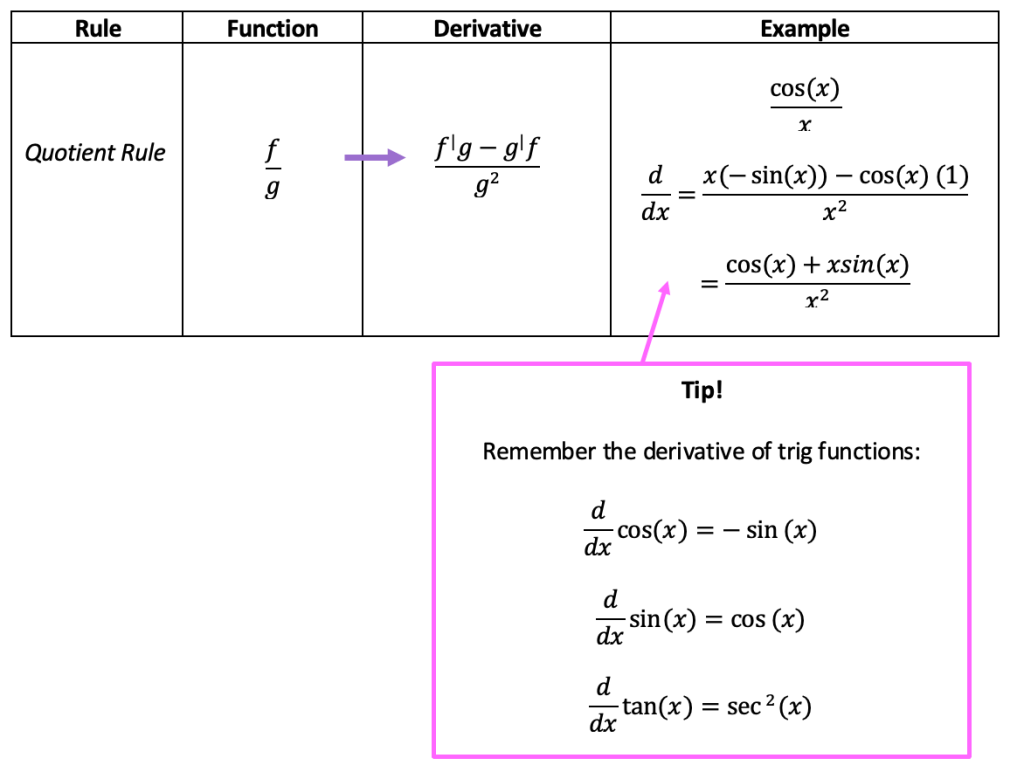
Quotient Rule:
Applying the quotient rule, will find the derivative of any two functions set up as a ratio. Be sure to notice any numbers or variables in the denominator that can be brought to the numerator (if that’s the case, can use the more friendly power rule).
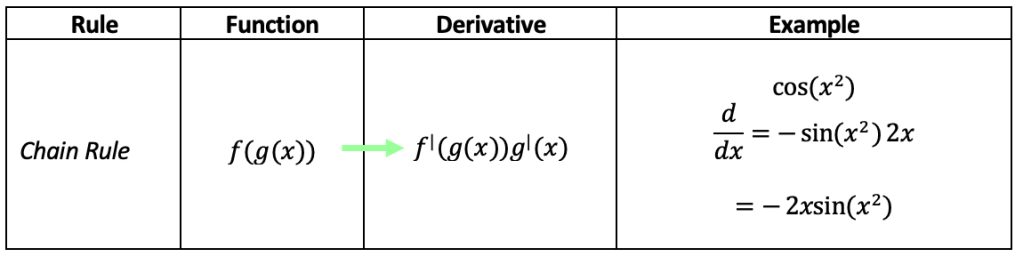
Chain Rule:
The chain rule allows us to find the derivative of nested functions. This is great for trigonometric functions and entire functions that are raised to an exponent.
Ready for some practice questions!? Check out the ones below to test your knowledge of derivatives!
Derivatives Practice Questions:
Find the derivatives for each function below.
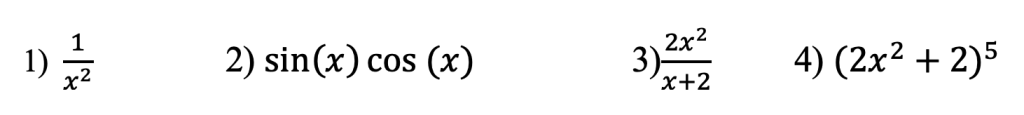
Solutions:
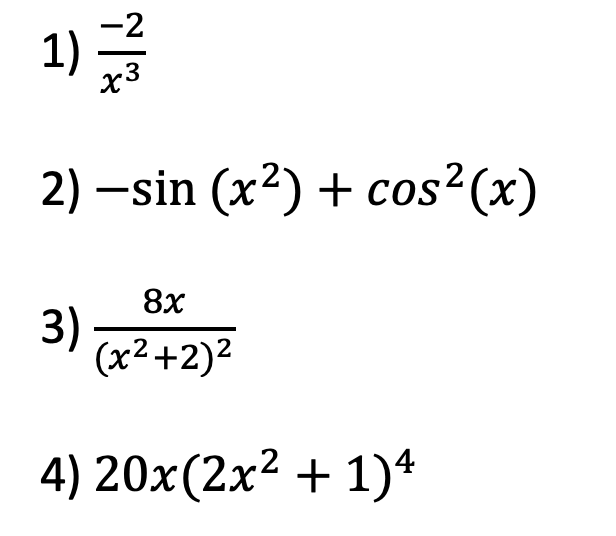
Still got questions? No problem! Don’t hesitate to comment with any questions or check out the video above. Happy calculating! ?
*Also, if you want to check out Rate of Change basics click this link here!
Facebook ~ Twitter ~ TikTok ~ Youtube





![Erratum for “An inverse theorem for the Gowers U^s+1[N]-norm”](https://azmath.info/wp-content/uploads/2024/07/2211-erratum-for-an-inverse-theorem-for-the-gowers-us1n-norm-150x150.jpg)

