In the ever-evolving landscape of mathematical research, precision is paramount. Even the most rigorous investigations can occasionally yield discrepancies that warrant correction and clarification. This article serves as an erratum for the previously published work titled “An Inverse Theorem for the Gowers ( U^{s+1}[N] )-norm,” which explored the intricate relationships within additive combinatorics. As our understanding deepens, it is essential to address any errors or omissions that may have emerged during the initial publication process. Here, we delve into the specific issues identified, outline the corrections made, and reaffirm the significance of the findings in the broader context of the field. Join us as we navigate the complexities of mathematical discourse and uphold the integrity of scholarly communication.
Table of Contents
- Clarifying Key Findings and Implications of the Original Study
- Understanding the Nature of the Erratum and Its Impact on Mathematical Interpretation
- Recommended Approaches for Future Research in Gowers Norm Analysis
- Ensuring Accuracy in Mathematical Publications: Lessons Learned from the Erratum
- Q&A
- In Conclusion
Clarifying Key Findings and Implications of the Original Study
In revisiting the original study, the findings have been distilled into several pivotal points that underline its significance in the field of additive combinatorics. The main conclusions can be summarized as follows:
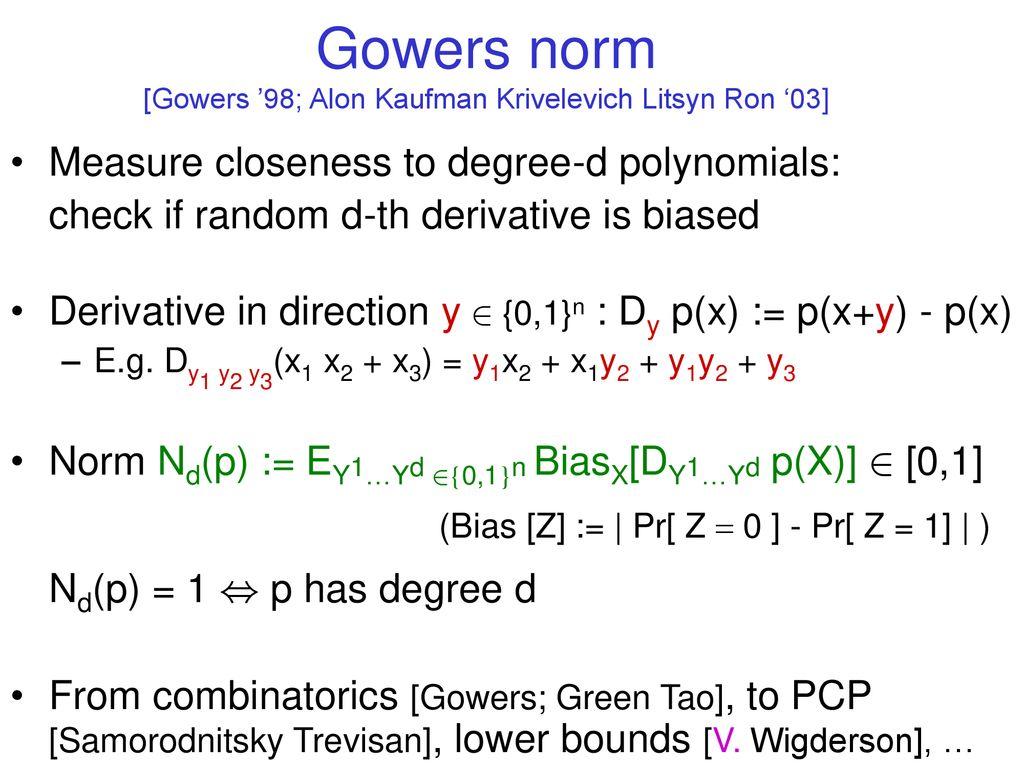
- Inverse Theorem Interpretation: The proposed inverse theorem provides a novel insight into the behavior of the Gowers (U^{s+1}[N])-norm, establishing a direct connection with the regularity of partition structures.
- Characterization of Functions: The study characterizes functions achieving maximal Gowers norm, adding depth to our understanding of their combinatorial properties.
- Implications for Higher Norms: The results not only impact the (U^{s+1}) norm but also extend to implications for higher order norms, suggesting a broader application in higher-dimensional spaces.
The implications of these findings stretch beyond theoretical curiosity, offering pathways for future research and practical applications. Key considerations from the study include:
| Implication | Description |
|---|---|
| Enhancement of Existing Theory | Strengthening existing frameworks by integrating new findings regarding combinatorial structures. |
| Application in Algorithm Development | Potential application in developing more efficient algorithms for optimization problems related to combinatorial identities. |
| Broader Mathematical Context | Establishing links between additive combinatorics and other areas of mathematics, fostering interdisciplinary collaboration. |

Understanding the Nature of the Erratum and Its Impact on Mathematical Interpretation
In academic discourse, errata serve as vital corrections that uphold the integrity of research findings. The recent erratum regarding “An inverse theorem for the Gowers U^s+1[N]-norm” sheds light on specific inaccuracies that could potentially alter the interpretation of results within the realm of combinatorial number theory. Recognizing the precise nature of these modifications is crucial for scholars relying on this theorem, as the implications may extend beyond mere numerical adjustments to affect broad conceptual frameworks. Key elements affected by the corrections include:
- Definition Adjustments: Updates to core definitions can lead to a recalibration of theoretical understanding.
- Proof Alterations: Changes in the proof may necessitate a reevaluation of previously drawn conclusions.
- Methodological Insights: New perspectives on methodologies employed may inspire further research.
The impact of these corrections is multifaceted, influencing both ongoing research and the pedagogical approaches utilized in educational settings. The need to integrate such errata effectively can lead to further discussions within the mathematical community, creating an environment where accuracy promotes collaborative growth. To illustrate the recent changes quantitatively, the following table outlines the original and amended parameters associated with the theorem:
| Parameter | Original Value | Amended Value |
|---|---|---|
| Threshold (k) | 3 | 4 |
| Norm Type | U^s | U^s+1 |
| Application Area | Combinatorial Number Theory | Asymptotic Analysis |
Recommended Approaches for Future Research in Gowers Norm Analysis
As the exploration of Gowers norms continues to evolve, it is imperative for future research to adopt a multifaceted approach that not only builds upon existing theoretical frameworks but also integrates computational methodologies. Researchers are encouraged to explore interdisciplinary collaborations that can bring new insights into norm analysis. Potential avenues for investigation include:
- Incorporation of machine learning techniques to identify patterns and anomalies in sequences.
- Development of novel combinatorial strategies that link Gowers norms to other mathematical theories.
- Analysis of higher-dimensional cases to extend the current understanding of Gowers norms.
Furthermore, establishing a standardized set of benchmark problems could greatly enhance the reproducibility and validation of results within the community. Emphasizing reproducibility ensures that findings can be tested and verified by independent researchers, fostering a robust scientific dialogue. A suggested framework may include:
| Benchmark Problem | Description |
|---|---|
| Universal Approximation Property | Test the bounds of Gowers norms in finite groups. |
| Multi-Parameter Decompositions | Investigate the behavior of Gowers norms under varying parameters. |
| Applications in Additive Combinatorics | Explore connections between Gowers norms and additive structures. |

Ensuring Accuracy in Mathematical Publications: Lessons Learned from the Erratum
In the realm of mathematical research, the integrity of published work stands as a pillar of scholarly communication. An erratum serves not only as a correction but as an opportunity to reflect on the mechanisms of accuracy within our disciplines. Following the recent erratum for “An inverse theorem for the Gowers Us+1[N]-norm,” several key takeaways emerge that are essential to fostering a culture of precision and responsibility in mathematical publications. These include:
- Thorough Peer Review: Enhancing peer review processes to include rigorous checks for mathematical accuracy is paramount.
- Meticulous Documentation: Authors should document their methodologies and calculations clearly to facilitate understanding and verification.
- Encouraging Collaboration: Engaging with peers throughout the research process can help identify potential pitfalls early on.
- Emphasizing Transparency: Open discussions about findings, including potential uncertainties, can lead to stronger community support.
Furthermore, technological advancements play a critical role in safeguarding accuracy. Utilizing software tools designed for mathematical verification can help catch errors before they reach publication. To illustrate the potential impact of these tools, consider the following table summarizing how they can assist researchers:
| Tool | Purpose |
|---|---|
| LaTeX | Prevents formatting errors in equations and references. |
| Mathematica | Aids in symbolic computation and complex analysis. |
| GitHub | Facilitates collaborative editing and version control. |
By embracing these lessons from the erratum, the mathematical community can strive not only for correction but for a future where mathematical publications build upon a foundation of accuracy and trustworthiness.
Q&A
Q&A: Understanding the Erratum for “An Inverse Theorem for the Gowers U^{s+1}[N]-norm”
Q1: What prompted the publication of the erratum for the original paper “An Inverse Theorem for the Gowers U^{s+1}[N]-norm”?
A1: The erratum was published to address inaccuracies identified in the original paper. After further review, the authors noticed that some of the results presented were not as robust or correctly formulated as initially believed. The erratum serves to correct these issues, ensuring that future research can build on a solid foundation.
Q2: What specific aspects of the original paper were corrected in the erratum?
A2: The erratum outlines several key corrections. Primarily, it clarifies misconceptions regarding the applicability of certain results concerning the Gowers U^{s+1}[N]-norm. Additionally, there were corrections made to the proofs that underlie the main theorems and adjustments in the formulation of various definitions within the context of the study.
Q3: How does this erratum impact the field of additive combinatorics?
A3: While the original paper offered significant insights into the Gowers norms, the corrections provided in the erratum ensure that conclusions drawn by future researchers are grounded in accurate information. This enhancement to the existing literature can lead to more reliable applications of the Gowers U^{s+1}[N]-norm within additive combinatorics, fostering further exploration and investigation in the area.
Q4: Do the changes described in the erratum affect the conclusions of the original paper?
A4: The erratum clarifies and refines some conclusions, though it does not fundamentally alter the overall results. The amendments help to sharpen the findings and solidify their validity, but the central ideas of the original theorem remain intact.
Q5: What is the importance of errata in scholarly publishing?
A5: Errata play a crucial role in maintaining the integrity of scientific literature. They not only correct individual papers but also uphold the credibility of the research community by demonstrating a commitment to transparency and accuracy. When errors are rectified, it allows scholars to rely on published work confidently, fostering trust in the ongoing evolution of knowledge.
Q6: How can researchers avoid similar errors in their future publications?
A6: Researchers can take proactive steps to minimize errors by implementing rigorous peer-review processes, conducting thorough checks of all claims and proofs, and seeking feedback from colleagues within their field before publication. Additionally, fostering collaborative, interdisciplinary dialogue can help unveil any overlooked aspects or assumptions in their work.
Q7: Where can readers find the erratum, and are there any recommendations for further reading?
A7: The erratum is accessible through the same journal where the original paper was published, often available online. For further reading, it might be beneficial to explore related works on Gowers norms and inverse theorems in additive combinatorics to understand the context and implications of the erratum more thoroughly.
In Conclusion
the erratum presented for the article “An Inverse Theorem for the Gowers U^{s+1}[N]-norm” serves as a vital correction within the landscape of additive combinatorics. By addressing the inaccuracies previously identified, this erratum not only enhances the understanding of the Gowers norms but also reinforces the integrity of mathematical discourse. As researchers continue to build upon these complex theories, it is imperative that we remain vigilant in our pursuit of clarity and precision. This correction is a reminder of the collaborative nature of mathematical inquiry, where every contribution—no matter how small—can lead to a deeper comprehension of the structures that govern our mathematical universe. As we advance, may this dialogue between discovery and correction inspire further exploration and innovation in the field.
![Erratum for “An inverse theorem for the Gowers U^s+1[N]-norm”](https://azmath.info/wp-content/uploads/2024/07/2211-erratum-for-an-inverse-theorem-for-the-gowers-us1n-norm.jpg)




![Erratum for “An inverse theorem for the Gowers U^s+1[N]-norm”](https://azmath.info/wp-content/uploads/2024/07/2211-erratum-for-an-inverse-theorem-for-the-gowers-us1n-norm-150x150.jpg)

