Title: Unraveling the Mysteries of Pi and the Arithmetic-Geometric Mean
In the world of mathematics, where numbers dance and equations weave intricate tapestries, two seemingly disparate concepts converge in a profound harmony: Pi and the Arithmetic-Geometric Mean (AGM). At first glance, Pi—a delightful and enigmatic number that represents the ratio of a circle’s circumference to its diameter—totals a staggering 3.14159 and continues infinitely, tantalizing mathematicians and enthusiasts alike with its infinite, non-repeating decimal sequence. Meanwhile, the AGM, a less-familiar but equally fascinating construct, emerges from the depths of number theory, playing a pivotal role in various mathematical applications, from approximating the square root of two to facilitating complex calculations in geometry.
As we explore the intriguing relationship between these two mathematical phenomena, we uncover a realm where circles meet averages, and precision dances with infinity. This article will delve into the foundational principles of both Pi and the AGM, illustrating how they interlink in the grand tapestry of mathematics. Join us on this journey as we seek to illuminate the profound connections that lie at the heart of these numerical wonders, and discover how they contribute to our understanding of mathematics and the universe itself.
Table of Contents
- Exploring the Intricacies of Pi and the Arithmetic-Geometric Mean
- The Historical Journey of Pi and Its Connection to Geometry
- Enhancing Computational Techniques Through Pi and AGM
- Practical Applications of Pi and AGM in Modern Mathematics
- Q&A
- Closing Remarks

Exploring the Intricacies of Pi and the Arithmetic-Geometric Mean
The enchantment of Pi is not merely in its infinite span of digits but also in its profound connections to other mathematical phenomena. At its core, Pi represents the ratio of a circle’s circumference to its diameter, captivating mathematicians and enthusiasts alike with its non-repeating, non-terminating nature. This irrational number has been a source of fascination throughout history, stimulating exploration in geometry, calculus, and even number theory. Among the many intriguing intersections within mathematics lies the **Arithmetic-Geometric Mean (AGM)**, a powerful computational tool that approximates circular measurements and contributes to various proofs and algorithms related to Pi.
The AGM provides an efficient method for calculating Pi, demonstrating an elegant interplay between arithmetic and geometric means. To understand this relationship, consider the following aspects:
- Convergence: The AGM is known for its rapid convergence, significantly speeding up calculations of Pi compared to traditional methods.
- Iterative Process: The procedure involves two sequences, one built on arithmetic means and the other on geometric means, culminating in an AGM value that can closely estimate Pi.
- Mathematical Applications: Beyond Pi, the AGM has implications in elliptic integrals and number theory, showcasing its versatility.
For those interested in the underlying mathematics, the following table summarizes the iterative steps to derive the AGM:
| Iteration | Arithmetic Mean (A) | Geometric Mean (G) |
|---|---|---|
| 1 | A1 = (a + b)/2 | G1 = √(ab) |
| 2 | A2 = (A1 + G1)/2 | G2 = √(A1G1) |
| 3 | A3 = (A2 + G2)/2 | G3 = √(A2G2) |
This convergence reveals the beauty of mathematics, offering a glimpse into the unity of circles and numbers through innovative techniques. The marriage of Pi and the AGM not only enriches our understanding of geometry but also exemplifies the creativity ingrained in mathematical exploration.
The Historical Journey of Pi and Its Connection to Geometry
The journey of Pi, that mysterious and fascinating constant, weaves through the annals of history, from ancient civilizations to modern mathematics. Cultures such as the Egyptians and Babylonians offered early approximations, grasping the essence of this ratio of the circumference of a circle to its diameter. **Archimedes of Syracuse** is often credited with one of the first rigorous calculations of Pi, employing a geometric method involving inscribed and circumscribed polygons. This laid the groundwork for future explorations, leading to more accurate computations over centuries, reflecting humanity’s ongoing quest to understand the infinite.
As mathematics evolved, Pi found itself inextricably linked to geometry and calculus. The **15th century** saw the introduction of symbolic representation, allowing for greater ease in manipulation and calculation. This was further deepened by the work of **Ludolph van Ceulen**, whose name is synonymously associated with Pi, as he calculated its value to 35 decimal places. The 18th century heralded the emergence of the **arithmetic-geometric mean (AGM)**, offering an innovative approach to computing Pi more efficiently. By iteratively averaging these two means, mathematicians were able to converge on the value of Pi with remarkable precision, showcasing its profound connection to geometric principles.
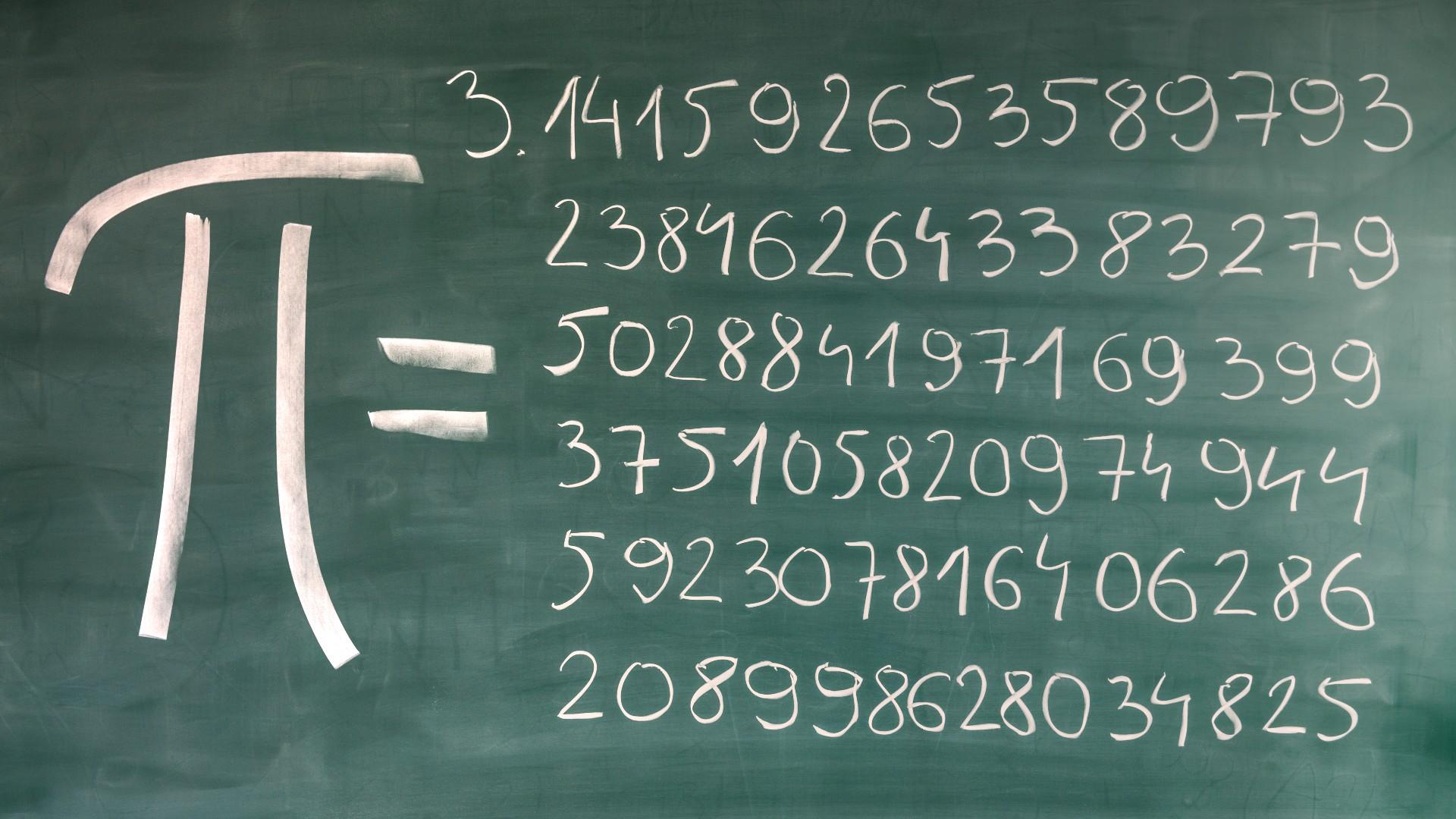
Enhancing Computational Techniques Through Pi and AGM
Throughout history, the quest to understand the digits of π has led to remarkable breakthroughs in computational techniques. The relationship between π and the Arithmetic-Geometric Mean (AGM) is a perfect illustration of this synergy. By integrating the repetitive process of averaging both arithmetic and geometric means, one can derive highly efficient algorithms for calculating π. This method not only enhances speed but also significantly boosts the accuracy of computations. Such advancements reveal connections between seemingly disparate areas of mathematics, demonstrating how improved algorithms can transform our understanding of numerical constants.
The methodologies rooted in AGM gear up computational efficiency in a variety of applications, including numerical analysis and cryptography. By employing the AGM approach, researchers and mathematicians can enhance the precision of π calculations that were once cumbersome. Key benefits include:
- Increased Convergence: AGM-based algorithms converge faster than traditional methods.
- Reduced Complexity: The AGM process simplifies the computational complexity involved in numerical approximations.
- Broader Applications: These techniques extend beyond pure mathematics into fields such as physics and engineering.

Practical Applications of Pi and AGM in Modern Mathematics
The concepts of Pi and the Arithmetic-Geometric Mean (AGM) possess remarkable applications in fields ranging from computer science to engineering. **Pi**, a mathematical constant derived from the ratio of a circle’s circumference to its diameter, plays a crucial role in algorithms that require precision in calculations involving circular and spherical geometries. For instance, in graphical rendering and simulations in computer games, the accurate depiction of curved surfaces is highly reliant on Pi. Similarly, **AGM** emerges as a vital tool in numerical approximations, notably in calculating elliptic integrals which have implications in areas such as cryptography and signal processing.
In practical scenarios, the synergy between Pi and AGM can be harnessed to optimize performance in various computational tasks. Some notable applications include:
- Algorithm Efficiency: Leveraging AGM for faster convergence in calculating values involving Pi.
- Signal Processing: Utilizing elliptic integrals for analyzing periodic signals with precision.
- Computer Graphics: Enhancing rendering techniques for more realistic animations.
Moreover, the integration of Pi and AGM methods opens pathways for innovative computational frameworks. The following table showcases a few algorithms that utilize both concepts:
| Algorithm Name | Application | Key Feature |
|---|---|---|
| Gauss-Legendre | Numerical Integration | Uses AGM for π approximation |
| Fast Fourier Transform | Signal Analysis | Applies properties of Pi |
| Iterative Solvers | Engineering Simulation | Incorporates AGM for speed |
Q&A
Q&A: Exploring the Intricacies of Pi and the Arithmetic-Geometric Mean (AGM)
Q: What is Pi and why is it considered so special in mathematics?
A: Pi (π) is an irrational number that represents the ratio of a circle’s circumference to its diameter. Its value starts as 3.14159 and continues infinitely without repeating. Its special status in mathematics arises from its ubiquitous appearance in various formulas across geometry, physics, and engineering. Pi is not only symbolically significant but also offers practical applications in calculating areas and volumes of circular shapes, making it a cornerstone of mathematical study.
Q: Can you explain what the Arithmetic-Geometric Mean (AGM) is?
A: The Arithmetic-Geometric Mean (AGM) is a two-variable mean used in mathematics that combines both arithmetic and geometric means. For two positive numbers, (a) and (b), the arithmetic mean is simply ((a + b) / 2) while the geometric mean is (sqrt{ab}). The AGM is derived through an iterative process where the arithmetic and geometric means are calculated repeatedly until they converge to a common value. This mean is particularly notable in multiple branches of mathematics, including number theory and approximation.
Q: How are Pi and the AGM connected?
A: The connection between Pi and the AGM stems from the AGM’s ability to provide extremely accurate approximations of elliptic integrals, where Pi frequently emerges. One famous result states that (pi) can be derived using the AGM through the relationship involving elliptic integrals of the first kind. More intriguingly, the AGM approach allows for computationally efficient ways to calculate Pi, showcasing the deep interrelationship between geometric concepts and numerical methods.
Q: Why is the AGM method considered significant for computing Pi?
A: The AGM method is significant for computing Pi due to its rapid convergence properties. Using this method, the number of correct digits of Pi can increase exponentially with each iteration. This makes the AGM not only a theoretical tool but also a practical one for those engaged in high-precision calculations. Its efficiency and elegance have made it a favorite among mathematicians and computer scientists alike who seek to explore the depths of Pi’s infinite nature.
Q: Are there any historical figures associated with the study of Pi and the AGM?
A: Yes, several historical figures have played pivotal roles in the study of Pi and the AGM. Archimedes is often celebrated for his early work on approximating Pi through inscribed and circumscribed polygons. In contrast, the AGM concept was formalized by mathematicians such as Carl Friedrich Gauss and later emphasized by mathematicians in the 19th and 20th centuries, including the legendary mathematician John von Neumann. Their contributions have laid the groundwork for modern explorations into these fascinating areas.
Q: How does understanding Pi and the AGM influence modern science and technology?
A: Understanding Pi and the AGM has profound implications for modern science and technology. In fields such as engineering, physics, and computer science, accurate calculations involving circles and cyclic phenomena are crucial. The AGM’s efficient methods for computing constants like Pi enable advancements in numerical simulations, graphical representations, and even quantum computing. As we continue to explore the complexities of mathematics, Pi and the AGM serve as reminders of the beauty and utility inherent in mathematical principles.
This Q&A offers a glimpse into the captivating interplay between Pi and the AGM, revealing their significance that stretches from ancient history to contemporary applications.
Closing Remarks
the relationship between Pi and the Arithmetic-Geometric Mean is a testament to the beauty and interconnectedness of mathematics. As we have explored, the AGM provides a fascinating pathway to approximating Pi, showcasing the intricate dance between geometry and arithmetic. This profound connection not only enriches our understanding of Pi but also highlights the elegance inherent in mathematical discovery. As you venture forth, may you carry with you a renewed appreciation for the surprising ways in which numbers intertwine, beckoning you to delve deeper into the enigmatic realms of mathematics. After all, in the pursuit of knowledge, even the simplest of numbers can unveil the most profound truths.





![Erratum for “An inverse theorem for the Gowers U^s+1[N]-norm”](https://azmath.info/wp-content/uploads/2024/07/2211-erratum-for-an-inverse-theorem-for-the-gowers-us1n-norm-150x150.jpg)

