Fashions and Delving Deeper Into Division

Fashions and Delving Deeper Into Division

 

Final month, we unpacked the long-division process taught in American Ok-6 lecture rooms, tied it into the usual “lengthy multiplication” algorithm, mentioned points with the lack of info traded for a rise in pace and effectivity, and in addition discover some different strategies for doing lengthy division.

 

What was taken with no consideration was that we knew what division “is” (I exploit scare citation marks right here advisedly: it’s harmful to attempt to be too restrictive concerning the underlying which means of “easy, elementary” mathematical operations, and I achieve this right here with out wishing to counsel that what I’m providing is or may presumably be definitive). My sense is that to a big extent, Ok-6 lecturers have at finest a really restricted grasp of what division means, notably when prolonged to the integers. This piece proposes to assist flesh out that understanding, notably for individuals who have struggled with the requisite concepts or not given the matter a lot consideration.

 

First, it’s affordable to state that division is the inverse operation for multiplication (I like to recommend avoiding or minimizing using “reverse” on this context), in the identical sense that subtraction is the inverse operation for addition. In truth, it’s by no means uncommon to outline subtraction as “including the inverse (or damaging)”; equally, we could outline division as “multiplying by the inverse (or reciprocal).” Nevertheless, some warning is required. If youngsters haven’t discovered about integers, lecturers must limit subtraction to instances of a – b the place b ≤ a. This raises points about whether or not to state that “You possibly can’t take away a much bigger quantity from a smaller quantity,” which is fake as soon as college students find out about damaging numbers. I like to recommend stating one thing alongside the traces of, “Given the numbers you realize about to date, questions like “What’s            3 – 5?” don’t have a solution; nonetheless, you’ll find out about numbers that permit us to reply questions like that in a number of years.”

 

Equally, earlier than they’ve entry to the rational numbers, questions like 16 ÷ 5 = ? must be answered utilizing remainders, and one thing alongside the traces of 3 ÷ 7 =? won’t make a lot sense in any respect! Stating flatly, “You possibly can’t divide a smaller quantity by a much bigger one” is just not solely deceptive but additionally leaves college students and lecturers alike in a critical quandary when confronted with dividing -6 by -3. Does nobody educating Ok-6 understand that whereas the reply is POSITIVE 2, the issue shouldn’t be doable inside the integers if that proscription about division is true?

 

Actual-World Fashions for Division

It’s not my competition that college students study arithmetic higher if they’ll floor it of their real-world experiences, or that they’ll get pleasure from arithmetic extra if they’ll relate it to their on a regular basis concrete experiences. That may nicely be true for some youngsters and irrelevant for others. Be that as it could, I imagine lecturers profit from having numerous fashions at their disposal as they educate wide-ranging college students arithmetic. And good fashions received’t take away from understanding so long as lecturers perceive and talk to their costs that fashions are methods to assist understanding the arithmetic; they don’t seem to be the arithmetic itself.

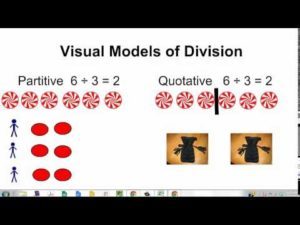
The 2 predominant fashions for division in elementary faculty arithmetic are the partitive (aka, fair-share) mannequin and the quotitive (measurement) mannequin.

Most younger youngsters have a fairly good intuitive/experiential understanding of what truthful shares are by the point division is launched in class, even when they don’t seem to be all that comfy with formal arithmetic operations (there’s a great deal of analysis with youngsters in Brazil and elsewhere that means many youngsters who work with cash in market stalls and different non-school settings develop facility with difficult calculations that aren’t mirrored of their faculty efficiency with arithmetic).

 

Given a hard and fast variety of gadgets, x (say, gummy bears), and a hard and fast variety of associates ok (together with herself), college students have efficient methods to find out how a lot every individual will get, q (share dimension), and what number of are left over, r (the rest) if any. What will get executed with the rest varies, after all. Native customs are usually not standardized.

 

What most youngsters know is that, the rest apart and assuming that each one gummy bears are equally fascinating, fair-sharing requires that every youngster will get the identical variety of bears. (Nevertheless, watch out for those who’re attempting to determine if given youngsters actually get the “fair-share” thought sufficient to attach it precisely with fractions (i.e., rational numbers). It’s commonplace for youthful youngsters sharing a sweet bar with one different individual to ask for “the larger half.” That makes excellent sense so long as we’re utilizing odd English somewhat than mathematical terminology.) A typical technique for determining the quotient is to distribute one bear at a time to every individual, repeating the method till there are not any extra gummies or not sufficient for everybody to get a further bear.

 

Observe that within the partitive mannequin of division, there’s a mounted whole/entire, x, a hard and fast variety of shares, ok, and an unknown share dimension, q, which is to be decided. For simplicity, we’ll not focus on how the rest, r, is “disposed of. “

 

With the quotitive mannequin, there’s once more a hard and fast whole/entire, x, however the dimension share is set, and what’s unknown is what number of shares/teams of this dimension could be made earlier than exhausting the availability or not having sufficient left to make one other group.

 

A typical scenario for quotitive division is cooking, the place there may be 12 cups of flour, and a cookie recipe that requires 1 1/3 cups of flour per batch. The query would now be, “What number of full batches of cookies could be made with 12 cups of flour?” (Assumed for this instance is that there are not any different constraints: ample quantities of all different components can be found).

 

Typically, it is a harder scenario for a lot of college students (and lecturers) to grapple with. Analysis has indicated, for instance, that many elementary lecturers and instructor training college students have an actual wrestle writing phrase issues that contain division by correct fractions. Requested to put in writing a ‘actual world downside’ that will be solved by dividing by ½, a big variety of these requested will as an alternative present one which represents division by 2, even when the dividend and divisor are explicitly offered in writing.

 

Modeling Division With Integers

Contemplate how partitive and quotitive fashions apply to signed quantity division, conserving in thoughts, too, that multiplication of actual numbers and their subsets is commutative, however division is just not.

 

For integers p, q, r, with r ≠ 0, take into account what occurs for numerous mixtures of p & q being optimistic or damaging. With p & q each optimistic, we are able to simply think about each partitive and quotitive division scenario and have already got talked about such examples.

 

If p & q are each damaging, say -12 and -3, we are able to ask meaningfully, “Into what number of teams of dimension -3 can we divide a complete of -12?” The reply, optimistic 4, is sensible arithmetically, nevertheless it will also be seen as distributing a debt of damaging $12 into equal teams of dimension damaging $3, then asking what number of companions can be wanted to soak up the debt equally. That is an instance of quotitive division.

 

If p is damaging and q is optimistic, we are able to additionally make a significant mannequin. Let’s say that p is once more –12 and that q is 3: then we would ask, “How a lot debt should every of three companions take to cowl a debt of $12?” Right here, the reply, -4, is sensible as a result of we’re speaking about sharing a hard and fast debt into a hard and fast variety of teams and every share accommodates the identical damaging variety of {dollars}. The mannequin is partitive.

 

Now, what occurs when p is optimistic and q is damaging? Can we make a wise partitive or quotitive mannequin? A little bit of thought means that we can not. We will’t have a partitive mannequin with a damaging divisor as a result of a damaging variety of teams merely is not sensible in the actual world. Alternatively, a measurement mannequin doesn’t work both. Making an attempt to divide a optimistic whole into shares of damaging dimension received’t fly.

 

Nonetheless, such computations pose no precise issue: 12 ÷ -3 = -4 and that is per our notions concerning the relationship between division and multiplication, since -4 • -3 = 12 Moreover, the principles college students are taught about signed-number multiplication and division maintain up: no contradiction is launched into arithmetic thereby. We should always all be joyful.

 

However what about our good fashions? The reply is, they break down right here. And maybe that’s a great factor. Arithmetic doesn’t rely upon a correspondence with the “actual world.” It depends upon logical consistency from the objects, guidelines for working with them, and the legal guidelines of reasoning. If we don’t arrive at contradictions, we’re joyful. Discovering a number of fashions or metaphors to assist understanding could also be fascinating, however it’s not vital.

 

So college students who’re in upper-elementary or center faculty who’re studying to consider sense making with signed-number division ought to have an opportunity to play and grapple with these points. In lots of instances, they need to be capable of deal with the extra summary thought of division as multiplication by the reciprocal. Ultimately, we wish all college students to have the ability to suppose extra abstractly when it comes to mathematical objects and guidelines for working with them. The interaction between fashions and arithmetic is ongoing even because the abstraction is ramped up, however fashions are a sort of scaffolding a lot of the time that we must be ready to desert when vital or handy, and the shortcoming to discover a mannequin for a specific little bit of arithmetic shouldn’t be an insuperable barrier to tackling it.