Growing Math Instinct

At present Kalid Azad launched a premium version of his well-received Math Higher Defined e-book.

This model new version (accessible for a restricted time for simply $47) boasts a screencast for every of the 12 chapters within the e book, in addition to a 3-part interview about growing your mathematical instinct with Scott H Younger, who has written extensively about examine methods. The bundle additionally features a PDF model of the e book, a worksheet, and slides/picture as a zipper file.

We have been supplied early entry to the fabric, and have discovered the e-book + screencasts combo to be extremely efficient in conveying elementary mathematical ideas that may serve you nicely for years to come back.

To have fun the announcement, and as a Math Weblog unique, immediately we’re publishing an excerpt from the e book right here. It covers learn how to develop math instinct and the nice significance this talent performs within the area of arithmetic. Get pleasure from!

Our preliminary publicity to an concept shapes our instinct. And our instinct impacts how a lot we get pleasure from a topic. What do I imply?
Suppose we need to outline a “cat”:

Caveman definition: A furry animal with claws, tooth, a tail, 4 legs, that purrs when pleased and hisses when offended. . .
Evolutionary definition: Mammalian descendants of a sure species (F. catus), sharing sure traits. . .
Trendy definition: You name these definitions? Cats are animals sharing the next DNA: ACATACATACATACAT. . .

The trendy definition is exact, positive. However is it the most effective? Is it what you’d train a toddler studying the phrase? Does it give higher perception into the “catness” of the animal? Probably not. The trendy definition is helpful, however after getting an understanding of what a cat is. It shouldn’t be our start line.

Sadly, math understanding appears to observe the DNA sample. We’re taught the trendy, rigorous definition and never the insights that led as much as it. We’re left with arcane formulation (DNA) however little understanding of what the concept is.

Let’s method concepts from a unique angle. I think about a circle: the middle is the concept you’re finding out, and alongside the skin are the details describing it. We begin in a single nook, with one truth or perception, and work our approach round to develop our understanding. Cats have frequent bodily traits results in Cats have a standard ancestor results in A species could be recognized by sure parts of DNA. Aha! I can see how the trendy definition advanced from the caveman one.

However not all beginning factors are equal. The appropriate perspective makes math click on — and the mathematical “cavemen” who first discovered an concept typically had an enlightening viewpoint. Let’s discover ways to construct our instinct.

1.1 What’s a Circle?

Time for a math instance: How do you outline a circle?

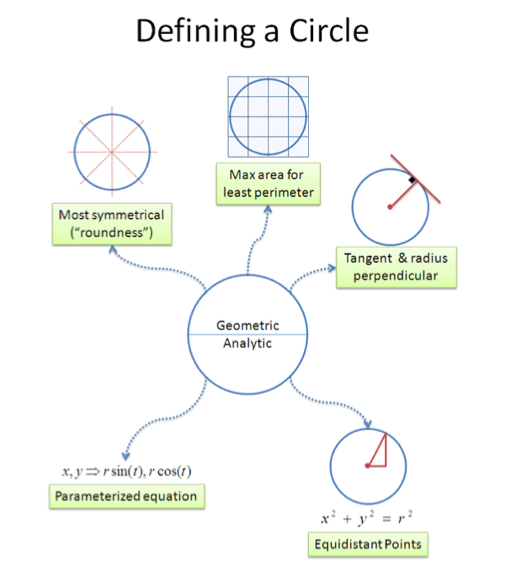
There are seemingly numerous definitions. Right here’s just a few:

Essentially the most symmetric 2-d form potential
The form that will get probably the most space for the least perimeter (the isoperimeter property)
All factors in a aircraft the identical distance from a given level (drawn with a compass, or a pencil on a string)
The factors (x,y) within the equation x² + y² = r² (analytic model of the geometric definition above)
The factors within the equation r · sin(t),r · cos(t), for all t (actually analytic model)
The form whose tangent line is at all times perpendicular to the place vector (bodily interpretation)

The checklist goes on, however right here’s the important thing: the details all describe the identical concept! It’s like saying 1, one, uno, eins, “the answer to 2x + 3 = 5” or “the variety of noses in your face” — simply totally different names for the concept of unity.

However these preliminary descriptions are essential — they form our instinct. As a result of we see circles in the true world earlier than the classroom, we perceive their “roundness”. It doesn’t matter what fancy equation we see (x² + y² = r²), we all know deep inside {that a} circle is spherical. If we graphed that equation and it appeared sq., or lopsided, we’d know there was a mistake.

As youngsters, we be taught the caveman definition of a circle (a very spherical factor), which provides us a cushty instinct. We will see that each level on our “spherical factor” is identical distance from the middle. x² + y² = r² is the analytic approach of expressing that truth (utilizing the Pythagorean theorem for distance). We began in a single nook, with our instinct, and labored our approach round to the formal definition.

Different concepts aren’t so fortunate. Can we instinctively see the expansion of e, or is it an summary definition? Can we understand the rotation of i, or is it a synthetic, ineffective concept?

1.2 A Technique For Growing Perception

I nonetheless need to remind myself concerning the deeper that means of e and that i — which appears as absurd as “remembering” {that a} circle is spherical or what a cat appears like! It needs to be the pure perception we begin with.

Lacking the large image drives me loopy: math is about concepts — formulation are only a solution to categorical them. As soon as the central idea is evident, the equations snap into place. Right here’s a technique that has helped me:

Step 1: Discover the central theme of a math idea. This may be tough, however attempt beginning with its historical past. The place was the concept first used? What was the discoverer doing? This use could also be totally different from our fashionable interpretation and utility.
Step 2: Clarify a property/truth utilizing the theme. Use the theme to make an analogy to the formal definition. Should you’re fortunate, you may translate the mathematics equation (x² + y² = r²) right into a plain-english assertion (“All factors the identical distance from the middle”).
Step 3: Discover associated properties utilizing the identical theme. After you have an analogy or interpretation that works, see if it applies to different properties. Generally it’ll, typically it received’t (and also you’ll want a brand new perception), however you’d be stunned what you may uncover.

Let’s attempt it out.

1.3 A Actual Instance: Understanding e

Understanding the quantity e has been a serious battle. e seems in all places in science, and has quite a few definitions, but not often clicks in a pure approach. Let’s construct some perception round this concept. The next part has a number of equations, that are merely methods to explain concepts. Even when the equation is gibberish, there’s a plain-english idea behind it. Right here’s just a few frequent definitions of e:

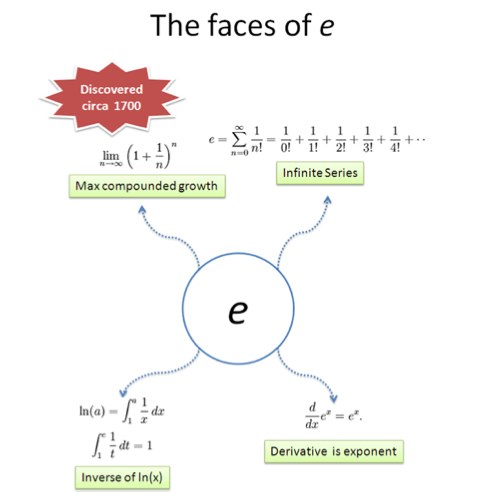
Step one is to discover a theme. Taking a look at e’s historical past, it appears it has some- factor to do with development or rates of interest. e was found when performing enterprise calculations (not summary mathematical conjectures) so “curiosity” (development) is a potential theme.

Let’s have a look at the primary definition, within the higher left. The important thing bounce, for me, was to appreciate how a lot this seemed just like the method for compound curiosity. In reality, it’s the curiosity method if you compound 100% curiosity for 1 unit of time, compounding as quick as potential. The chapter on e describes this interpretation.

Definition 1: Outline e as 100% compound development on the smallest increment potential.

Let’s have a look at the second definition: an infinite sequence of phrases, getting smaller and smaller. What may this be?

[tex]displaystyle e = frac{1}{0!} + frac{1}{1!} + frac{1}{2!} + frac{1}{3!} + ldots[/tex]

After noodling this over utilizing the theme of “curiosity” we see this definition reveals the parts of compound curiosity. Now, insights don’t come immediately — this perception would possibly strike after brainstorming “What may 1 + 1 + 1/2 + 1/6 + . . . ” symbolize when speaking about development?”

Properly, the primary time period (1 = 1/0!, remembering that 0! is 1) is your principal, the unique quantity. The following time period (1 = 1/1!) is the “direct” curiosity you earned — 100% of 1. The following time period (0.5 = 1/2!) is the amount of cash your curiosity made (“2nd-level curiosity”). The next time period (.1666 = 1/3!) is your “Third-level curiosity” — how a lot cash your curiosity’s curiosity earned!

Cash earns cash, which earns cash, which earns cash, and so forth — the sequence separates out these contributions (the chapter on e describes how Mr. Blue, Mr. Inexperienced & Mr. Crimson develop independently). There’s way more to say, however that’s the “growth-focused” understanding of that concept.

Definition 2: Outline e by the contributions each bit of curiosity makes.

Neato. Now to the third, shortest definition. What does it imply? As an alternative of considering “spinoff” (which turns your mind into equation-crunching mode), take into consideration what it means. The sensation of the equation. Make it your buddy.

[tex]displaystyle frac{d}{dx}Blah = Blah[/tex]

It’s the calculus approach of claiming “Your fee of development is the same as your present quantity”. Properly, rising at your present quantity can be a 100% rate of interest, proper? And by at all times rising it means you’re at all times calculating curiosity – it’s one other approach of describing constantly compound curiosity!

Definition 3: Outline e as at all times rising by 100% of your present worth.

Good — e is the quantity the place you’re at all times rising by precisely your present quantity (100%), not 1% or 200%.

Time for the final definition — it’s a difficult one. Right here’s my interpretation: As an alternative of describing how a lot you grew, why not say how lengthy it took?

Should you’re at 1 and rising at 100%, it takes 1 unit of time to get from 1 to 2. However when you’re at 2, and rising 100%, it means you’re rising at 2 items per unit time! So it solely takes 1/2 unit of time to go from 2 to three. Going from 3 to 4 solely takes 1/3 unit of time, and so forth.

The time wanted to grom from 1 to A is the time from 1 to 2, 2 to three, 3 to 4. . . and so forth, till you get to A. The primary definition defines the pure log (ln) as shorthand for this “time to develop” computation.

ln(a) is just the time to develop from 1 to a. We then say that e is the quantity that takes precisely 1 unit of time to develop to. Mentioned one other approach, e is is the quantity of development after ready precisely 1 unit of time!

Definition 4: Outline the time wanted to develop constantly from 1 to as ln(a). e is the quantity of development you may have after 1 unit of time.

Whablamo! These are 4 other ways to explain the mysterious e. As soon as we have now the core concept (“e is about 100% steady development”), the loopy equations snap into place — it’s potential to translate calculus into English. Math is about concepts!

1.4 What’s the Ethical?

In math class, we frequently begin with the final, most advanced concept. It’s no marvel we’re confused: we’re exhibiting college students DNA and anticipating them to see a cat. I’ve realized just a few classes from this method, and it underlies how I perceive and clarify math:

Seek for insights and apply them. That first intuitive perception may also help every thing else snap into place. Begin with a definition that is smart and “stroll across the circle” to search out others.
Be resourceful. Banging your head in opposition to an concept is not any enjoyable. If it doesn’t click on, come at it from totally different angles. There’s one other e book, one other article, one other one who explains it in a approach that is smart to you.
It’s alright to be visible. We consider math as inflexible and analytic — however visible interpretations are okay! Do what develops your understanding. Imaginary numbers have been puzzling till their geometric interpretation got here to gentle, a long time after their preliminary discovery. Taking a look at equations all day didn’t assist mathematicians “get” what they have been about.

Math turns into tough and discouraging once we concentrate on definitions over understanding. Keep in mind that the trendy definition is probably the most superior step of thought, not essentially the place to begin. Don’t be afraid to method an idea from a humorous angle — work out the plain-English sentence behind the equation. Completely satisfied math.

Order the e book and screencasts.

Disclaimer: The hyperlinks to Math Higher Defined comprise our referrer id.