The MRB fixed is the higher restrict level of the sequence of partial sums outlined by:
[tex]displaystyle S(x) = sum_{n=1}^{x}{(-1)^n n^frac{1}{n}}[/tex].
The aim of this text is to indicate that the MRB fixed is geometrically quantifiable. To “measure” the MRB fixed, we are going to take into account a set, sequence and alternating collection of the nth roots of n. Then we are going to evaluate the size of the sides of a particular set of hypercubes or n-cubes which have a content material of n. (The 2 phrases hypercubes and n-cubes will probably be used synonymously.)
Lastly we are going to have a look at the worth of the MRB fixed as a illustration of that comparability, of the size of the sides of a particular set of hypercubes, in items of dimension 1/ (items of dimension 2 occasions items of dimension 3 occasions items of dimension 4 occasions and so on.). For an arbitrary instance we are going to use items of size/ (time*mass* density*…).
A Countable Infinite Set
Think about, r, the set of roots of optimistic integers of the shape r = n^(1/n). In fact the weather of this set are of the shape x^(1/y). Nonetheless what is just not apparent is the geometric interpretation of x^(1/y). A minimum of so far as pure quantity valued (x and y)>0 are involved, x represents the content material of an n-cube and y represents its dimension. That could be a geometric interpretation of x^(1/y) so far as pure quantity valued (x and y)>0 are involved. As an illustration we take a dice of any given quantity and discover the size of one among its sides. Let’s suppose the quantity was 8 items^3. What can be the size of one among its sides? We would simply deduce that the size is 2. To verify this reply we merely assemble a dice of two linear items in size as in Diagram 1 and discover its quantity.
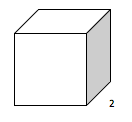
Diagram 1
The amount in items3 of the dice in Diagram 1 is certainly 2*2*2 = 8.
Now we have a look at the earlier sentence with x^(1/y) in thoughts. 8^(1/3) = 2 implies the quantity of the dice in Diagram 1 raised to the facility of the reciprocal of its dimension equals the size of one among its sides. That could be a geometric interpretation of x^(1/y) so far as pure quantity valued x and y > 0 are involved.
Open Query
What’s the geometric interpretation of x^(1/y) for all actual values of x,y > 0?
Sequence and Alternating Sequence
Now we are going to take into account the sequence of roots of optimistic integers of the shape r = {n^(1/n)} = {1^(1/1), 2^(1/2), 3^(1/3), …}. Then we are going to add the weather of r within the alternating collection
[tex]displaystyle L = sum_{n=1}^{infty}{(-1)^n r(n)} = sum_{n=1}^{infty}{(-1)^n n^frac{1}{n}}.[/tex]
In regards to the partial sums of L, we keep in mind
[tex]displaystyle S(x)= sum_{n=1}^{x}{(-1)^n n^frac{1}{n}}[/tex]
and we discover, S(x) is divergent as x goes to infinity. Nonetheless S(2x) and S(2x+1) are each convergent as x goes to infinity and the distinction between S(2x) and S(2x+1) additionally converges.
Particular Hypercubes
In geometric phrases: As in Diagram 2, we give every n-cube a content material equal to its dimension1,
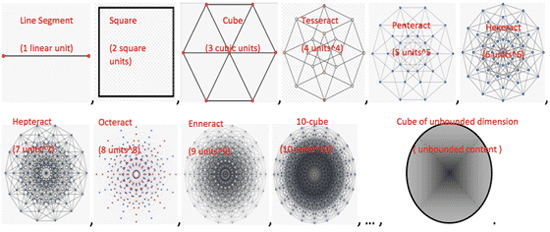
Diagram 2
in order that now we have a line phase of 1 linear unit, a sq. of two sq. items, and so forth.
The final dice within the diagram could also be simply an invention of the creativeness as a result of who has ever heard of a hypercube of unbounded dimension with unbounded content material? Moreover, there appears to be a paradox invoked when taking n-cubes as n->∞. Whereas n remains to be a quantity the content material of the n-cube or hypercube is outlined within the particular unit of alternative, whether or not the unit is inches, meters or what not and the ensuing size of a person edge can be outlined in the identical unit and is computed as proven above. “The [content] of the [n-]dice raised to the facility of the reciprocal of its dimension equals the size of one among its [edges].”
Nonetheless, once we arrived on the hypercube of unbounded dimension the place n is not a quantity, the assigned “unbounded content material” might be meant to be in items of toes, let’s say; whereas the ensuing size of a person edge is [tex]displaystyle lim_{u to infty}{u^frac{1}{u}} = 1[/tex] which might be in toes or every other unit of size as a result of there are the identical quantity of inches or meters or every other unit of size in infinity toes as there are toes. To keep away from the ensuing ambiguity we are going to have a look at the sequence of roots of optimistic integers of the shape r = {n^(1/n)} = {1^(1/1), 2^(1/2), 3^(1/3), …} as being analogous to the clopen interval [1, ∞).
A Sum of the Series
Above it is mentioned, “Add the elements of r in the alternating series
[tex]displaystyle L = sum_{n=1}^{infty}{(-1)^n r(n)} = sum_{n=1}^{infty}{(-1)^n n^frac{1}{n}}.[/tex]”
To indicate that geometrically we do the next: as in Diagram 3, on the y,z-plane line up an edge of every n-cube or hypercube. The numeric values displayed within the diagram are the partial sums of S(x) = S(2u) the place u is a optimistic integer:
[tex]displaystyle S(x)=sum_{n=1}^{x}{(-1)^n n^frac{1}{n}}.[/tex]
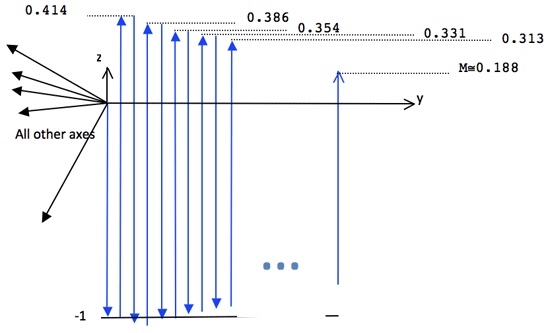
Diagram 3
Discover a directed line phase is moved from the origin down the (z or y=0)-axis. Then on the y=1δ axis one other one is moved up 2^(1/2) items. Then on the y=2δ axis one more one is moved down 3^(1/3) items, and so on. It doesn’t matter whether or not δ is one or every other actual worth; there nonetheless are an infinite variety of y-valued axes with matching directed line segments.
That is onerous to know; however we might say metaphorically that Diagram 3 is the trail alongside the items of a particle moved 1 inch down in 2^(1/2) seconds, shedding 3^(1/3) items of mass with density that will increase 4^(1/4) items and so on. The ensuing place and situation of the particle is represented by
[tex]displaystyle M = lim_{u to infty}{left ( sum_{n=1}^{2u}{(-1)^n n^frac{1}{n}} proper )}.[/tex]
The MRB Fixed
Because the dimension and the content material of a hypercube, each go to infinity now we have the next: First, in Diagram 2 the distinction between the size of an fringe of the hypercube with content material 2n+1, and an fringe of the hypercube with content material 2n, goes to the fixed worth,
[tex]displaystyle lim_{n to infty}{left ( (2n +1)^frac{1}{2n+1}-(2n)^frac{1}{2n} proper )} = 0.[/tex]
In order n goes to infinity, the size of an fringe of the hypercube with content material 2n and an fringe of the hypercube with content material 2n+1 change into nearer to being the identical. Second, in Diagram 3 an edge of every n-cube is organized on y-valued axes in such a means that
[tex]displaystyle sum_{n=0}^{infty}{left ( (2n +1)^frac{1}{2n+1}-(2n)^frac{1}{2n} proper )} = lim_{u to infty}{left ( sum_{n=1}^{2u}{(-1)^n n^frac{1}{n}} proper )}.[/tex]
M is the MRB fixed2.
A numerical approximation of M could be computed by the next summation
[tex]displaystyle sum_{n=1}^{infty}{(-1)^n left (n^frac{1}{n}-1 proper )}[/tex],
which sum converges3 (see Diagram 4), whereas
[tex]displaystyle sum_{n=1}^{infty}{(-1)^n n^frac{1}{n}}[/tex]
diverges4, as talked about above.
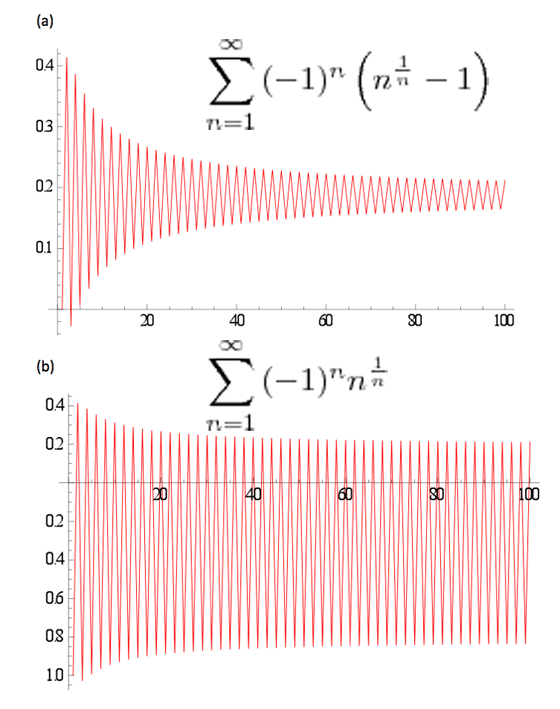
Diagram 4
One ought to use acceleration strategies when computing a numerical approximation of the MRB fixed as a result of it may be proven that one should sum a quantity within the order of 10^(n+1) iterations of (-1)^n*(n^(1/n)-1) to get n correct digits of the MRB Fixed. Nonetheless, utilizing a convergence acceleration of alternating collection algorithm of Cohen-Villegas-Zagier one can compute the primary 60 digits in solely 100 iterations5.
In Diagram 4a each the lim sup6 and the lim inf converge upon the MRB fixed, whereas in 4b solely the lim sup converges upon it with the lim inf converging upon MRB constant-1. The MRB fixed is Sloane’s On-Line Encyclopedia of Integer Sequences id:A0370777. Extra data, together with a quick however documented historical past, could be present in Wikipedia8.
Abstract
On reflection, the geometry used right here, notably in diagram3, is transdimensional and thus we discover it onerous to know via the earlier experiences of our senses. (To look at its geometry we used edges from hypercubes of many dimensions.) Nonetheless, contemplating the varied temporal-spatial dimensions that have an effect on our universe as proposed in some theories9 is there some significance to the MRB fixed in our every day lives? However, now we have seen that the worth of the MRB fixed is geometrically quantifiable; it’s the lim sup of the sequence that represents a particle touring alongside a directed line phase that’s moved 1 unit from the origin down the z-axis; on the y=1δ moved up 2^(1/2) items’ on the y=2δ axis moved down 3^(1/3) items and so on. Whether or not the items are theoretical (as in items of size/ (time*mass* density*…)) or proposed temporal-spatial dimensions, the ensuing z worth of the particle’s place and situation
[tex]displaystyle M = lim_{u to infty}{left ( sum_{n=1}^{2u}{(-1)^n n^frac{1}{n}} proper )}.[/tex]
This text is launched below the Artistic Commons Attribution-Share Alike 3.0 Unported license.
In regards to the writer
Marvin Ray Burns has indulged in math analysis as a pastime since 1994. Having had just one school course, most of his discoveries had been merely studying the fundamentals of math. He has cataloged lots of his early investigations at https://math2.org/mmb/search?question=Marvin. One in every of his concepts has served some goal within the math world; that’s the MRB fixed. Because the discovery of the MRB fixed, a minimum of one main arithmetic software program firm has discovered that it was helpful to repair issues discovered whereas computing the MRB fixed. One other main firm modified the performance of its sum operate in such a means as to have the ability to compute the digits of the MRB fixed shortly after its discovery. Mr. Burns has submitted just a few integer sequences based mostly on his explorations of the MRB fixed; see https://www.analysis.att.com/~njas/sequences/?q=A037077. A siding applicator by career, he presently takes varied undergraduate programs at IUPUI within the hopes of acquiring a level in pure math.
References
[1] https://www23.wolframalpha.com/enter/?i=n-cube
[2] S. R. Finch, Mathematical Constants, Cambridge, 2003, p. 450.
[3] https://mathworld.wolfram.com/MRBConstant.html
[4] https://mathworld.wolfram.com/notebooks/Constants/MRBConstant.nb
[5] https://arxiv.org/abs/0912.3844
[6] https://en.wikipedia.org/wiki/Upper_limit
[7] https://oeis.org/A037077
[8] https://en.wikipedia.org/wiki/MRB_constant
[9] https://arxiv.org/abs/hep-ph/9803466




![Erratum for “An inverse theorem for the Gowers U^s+1[N]-norm”](https://azmath.info/wp-content/uploads/2024/07/2211-erratum-for-an-inverse-theorem-for-the-gowers-us1n-norm-150x150.jpg)

