Math Games – building a foundation for mathematical reasoning – Thinking Mathematically

In 2001, the National Research Council, in their report Adding it up: Helping children learn mathematics, sought to address a concern expressed by many Americans: that too few students in our schools are successfully acquiring the mathematical knowledge, skill, and confidence they need to use the mathematics they have learned.

Developing Mathematical Proficiency
The potential of different types of tasks for student learning, 2017

As we start a new school year, I expect many teachers, schools and districts to begin conversations surrounding assessment and wondering how to start learning given students who might be “behind”. I’ve shared my thoughts about how we should NOT start a school year, but I wanted to offer some alternatives in this post surrounding a piece often overlooked — our students’ confidence (including student agency, ownership and identity). If we are truly interested in starting a year off successfully, then we need to spend time allowing our students to see themselves in the math they are doing… and to see their strengths, not their deficits.

[The] goal is to support all students — especially those who have not been academically successful in the past — to develop a sense of agency and ownership over their own learning. We want students to come to see themselves as intellectually capable and competent — not by giving them easy successes, but by engaging them as sense-makers, problem solvers, and creators of meaningful and important ideas.

MathShell – TRUMath, 2016

When we hear ideals like the above quote, what many of us see is as missing are specific examples. How DO we help our students gain confidence becomes a question most of us are left with. Adding It Up suggests that mathematical proficiency includes an intertwined mix of procedural fluency, conceptual understanding, strategic competence, adaptive reasoning and productive disposition. Which again sounds nice in theory, but in reality, these 5 pieces are not balanced in classroom materials nor in our assessment data. Not even close!

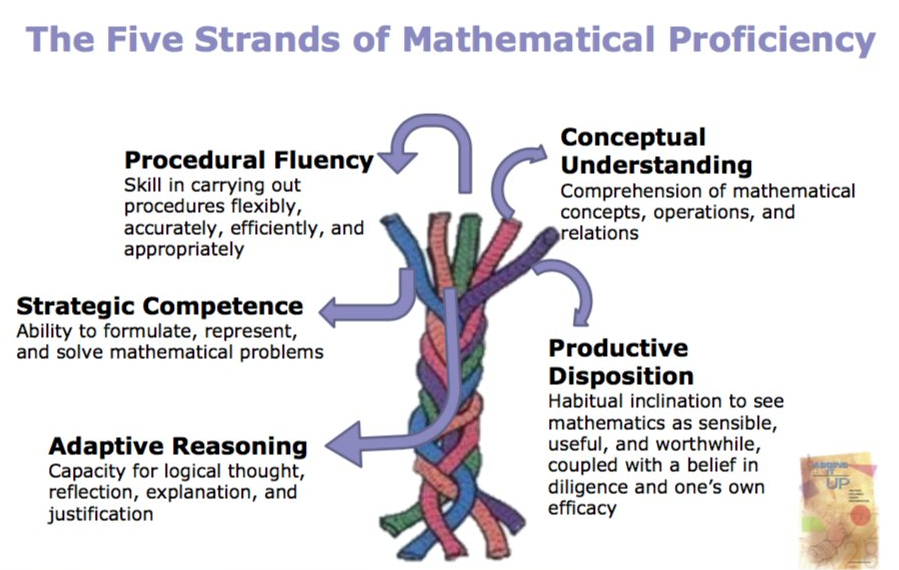
So, again we are left with a specific need for us to build confidence in our students. There is a growing body of evidence to support the use of strategy games in math class as a purposeful way to build confidence (including student agency, authority and identity).

To be helpful, I’d like to share some examples of possible strategy games that are appropriate for all ages. Each game is a traditional game from various places around the world.

*The above files are open to view / print. If you experience difficulties accessing, you might need to use a non-educational account as your school board might be restricting your access.

How to Play:

Each link above includes a full set of rules, but you might also be interested in watching a preview of these games (thanks to WhatDoWeDoAllDay.com)

A few things to reflect on:

• Some students have missed a lot of school / learning. Our students might be entering a new grade worried about the difficulty level of the content. Beyond content, what other aspects of learning math might be a struggle for our students? How might introducing games periodically help with these struggles?
• How do you see equity playing a role in all of this? Pinpointing and focusing on student gaps often leads to inequities in experiences and outcomes. So, how can the ideas above help reduce these inequities?
• One of the best ways to tackle equity issues is to expand WHAT we consider mathematics and expand WHO is considered a math person. How might you see using games periodically as a way for us to improve in these two areas?
• If you are distance learning, how might games be an integral part of your program? How do you see including games that are not related to content helpful for our students that might struggle to learn mathematics? (building confidence, social-emotional learning skills, community, students’ identities…)
• If you are learning in person this year, but can not have students working together, how might you adapt some of these strategy games?
• What might you notice as students are playing games that you might not be able to notice otherwise?
• How might we see a link between gaining confidence through playing strategy games and improvement in mathematical reasoning?
• Why do you think I choose the games above (I searched through many)? Hopefully you can see a benefit from seeing mathematics learning from various cultures.

If interested in more games and puzzles? Take a look at some of the following posts:

As always, I’d love to hear your thoughts.  Leave a reply here on Twitter (@MarkChubb3)