Greetings math peeps and welcome to another week of MathSux! In todays post we are going to explore how to find the legs of a right triangle when an altitude is drawn from the vertex to its hypotenuse. We are going to take this step by step on how to solve a problem like this. Although, I go over the long way to solve this problem, there is also short cut many people use called the “geometric means” which is also briefly mentioned in this post (under the Tip! section). Whichever method you choose, do what makes most sense to you! Happy calculating!
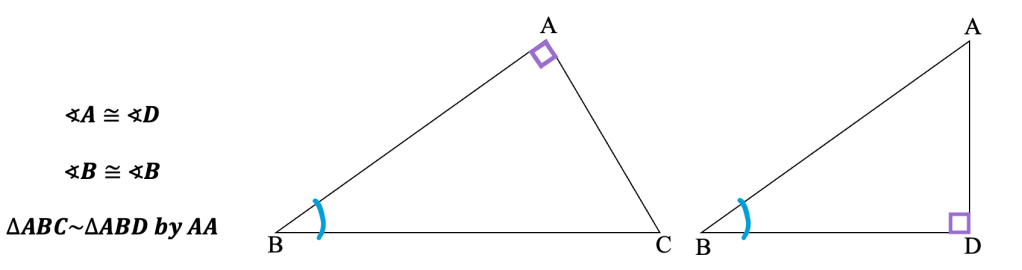
Similar Right Triangles (with Altitude drawn):
When two triangles have equal angles and proportionate sides, they are similar. This means they can be different in size (smaller or larger) but if they have the same angles and the sides are in proportion, they are similar! Triangles can be proven similar by AA, SAS, or SSS. For more on similar triangles, check out this post here.
There is a special type of scenario that happens with similar right triangles. When an altitude is drawn from the vertex of a right triangle, it forms two smaller triangles, which creates three right triangles that are similar to the original triangle, based on Angle Angle (AA). Check out the example to see how it works!
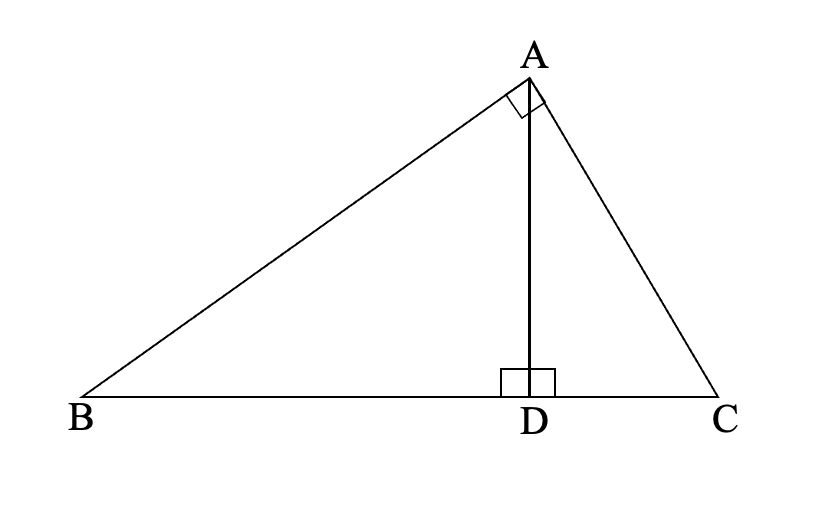
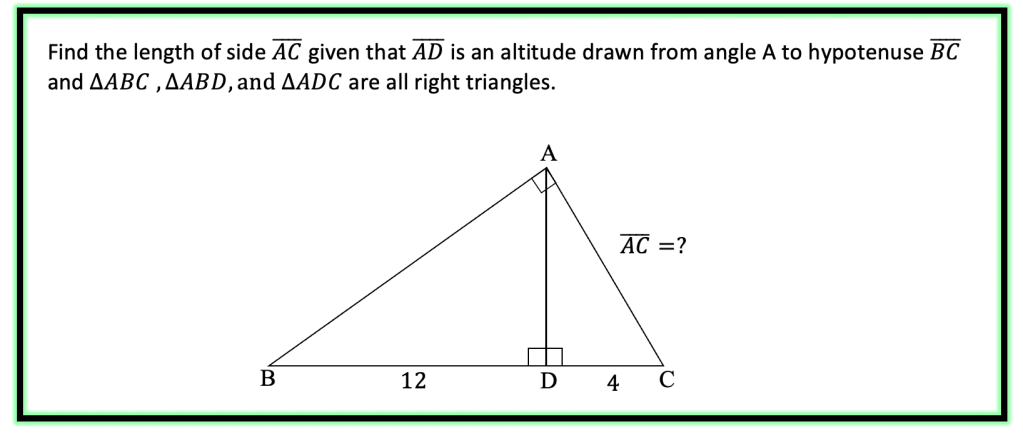
In triangle ABC, an altitude is drawn from angle A to its hypotenuse BC. Notice that this creates three 90º right triangles in total (ABC, ABD, and ADC).
Drawing an altitude created three total right triangles, broken out below:
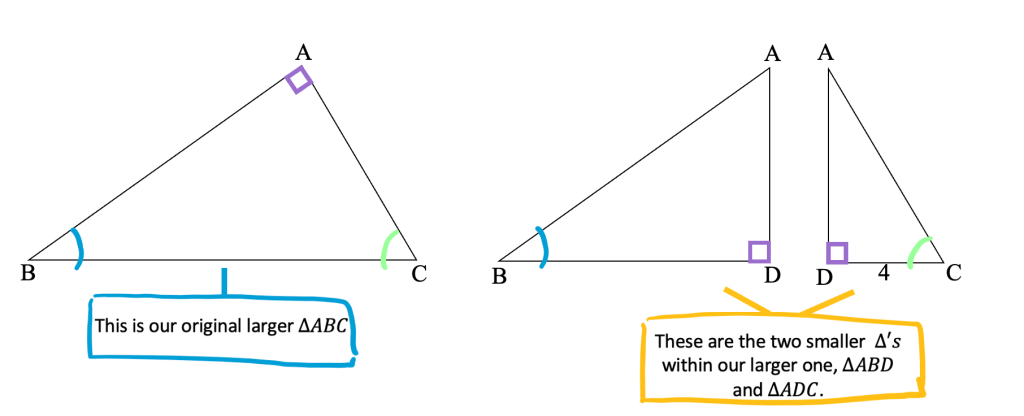
If you take a closer look, at the triangles above you’ll notice that these two new triangles (ABD and ADC) share an angle with the original bigger triangle ABC and a 90º angle. This makes each of the new triangles similar to the original triangle by AA. We can therefore say that:

How are the New Right Triangles Similar?
1) Triangle ABC ~ ADC
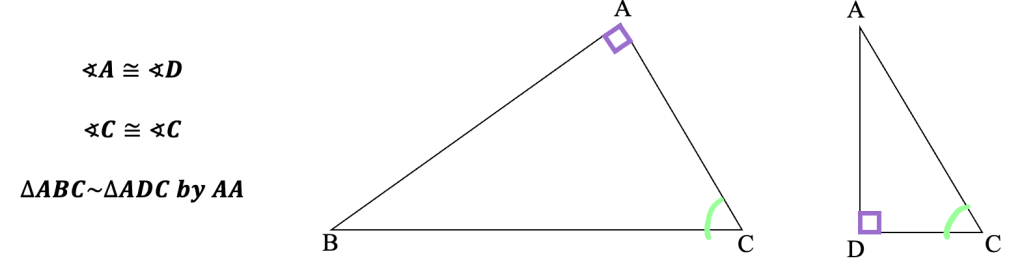
2) Triangle ABC ~ ADB
Ready for an Example? Lets check out the one below!
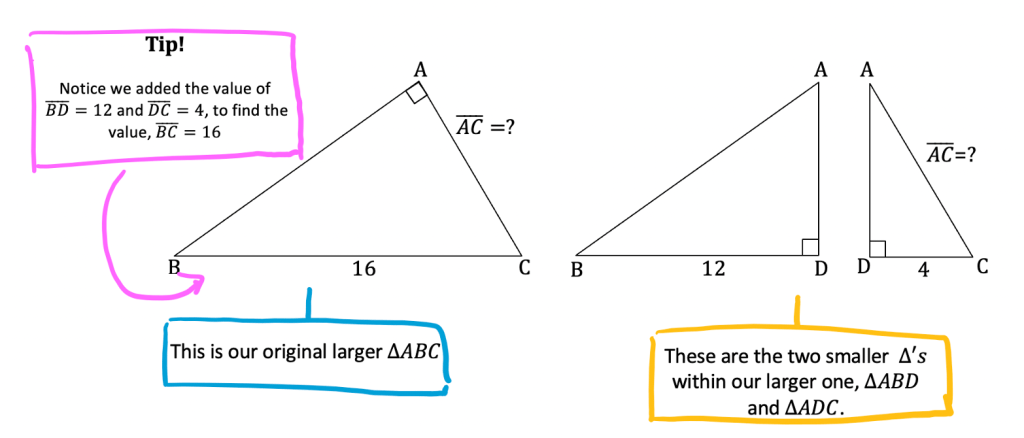
Step 1: To find the length of the missing legs of a right triangle, first, let’s separate each right triangle to see what we’re working with, along with the values of each length that was provided.
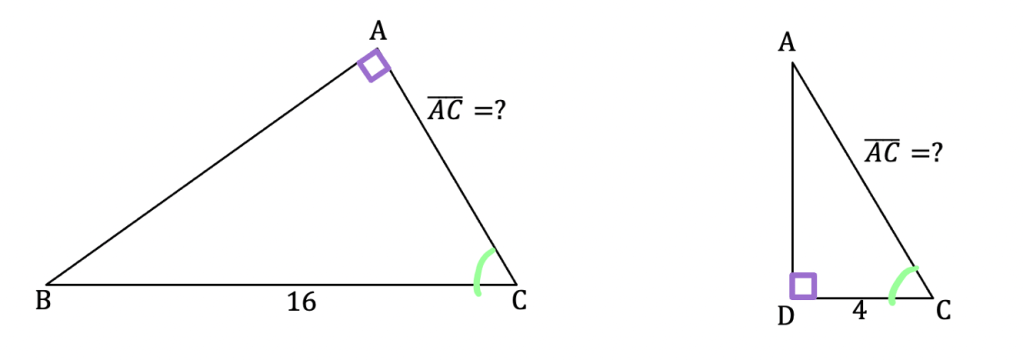
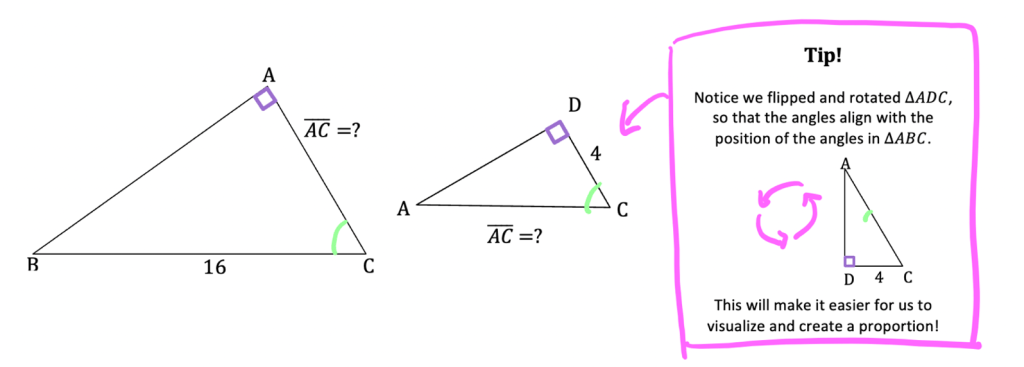
Step 2: We want to find the length of side AC, so let’s use the triangles whose sides include AC. This leads us to use triangles ABC and ADC.
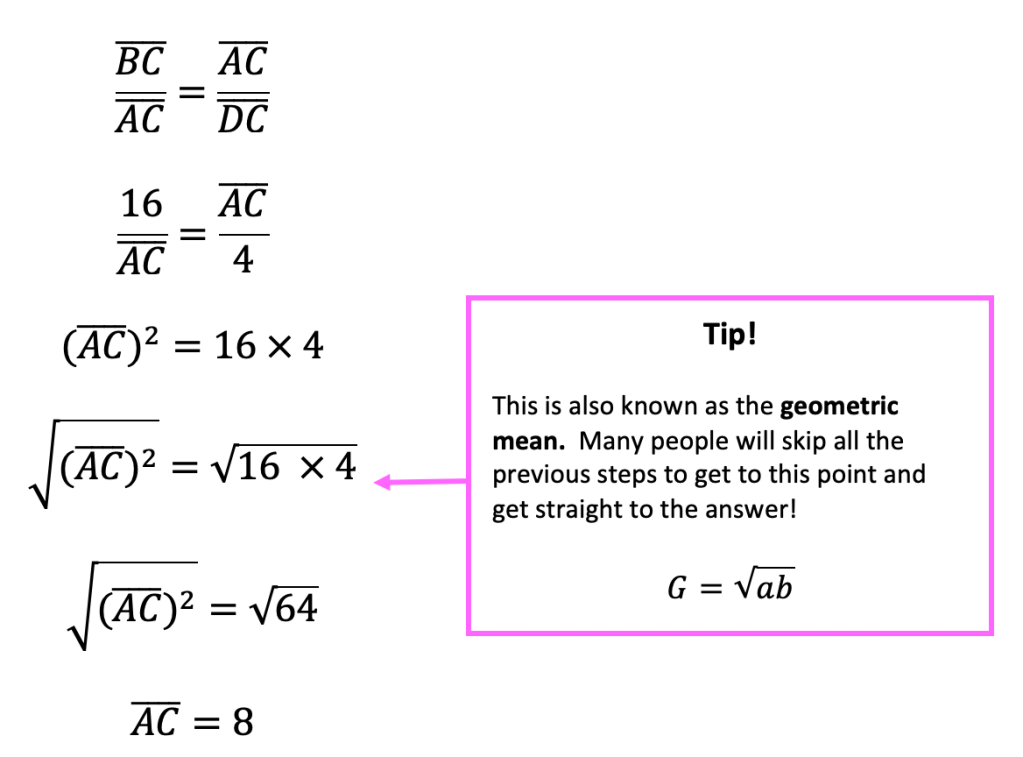
Step 3: Now, let’s set up our proportion to find our missing side. To make it easier for ourselves let’s first flip and rotate triangle ADC to line up with triangle ABC.

Think you’re ready to try some practice questions on your own? Check out the ones below!
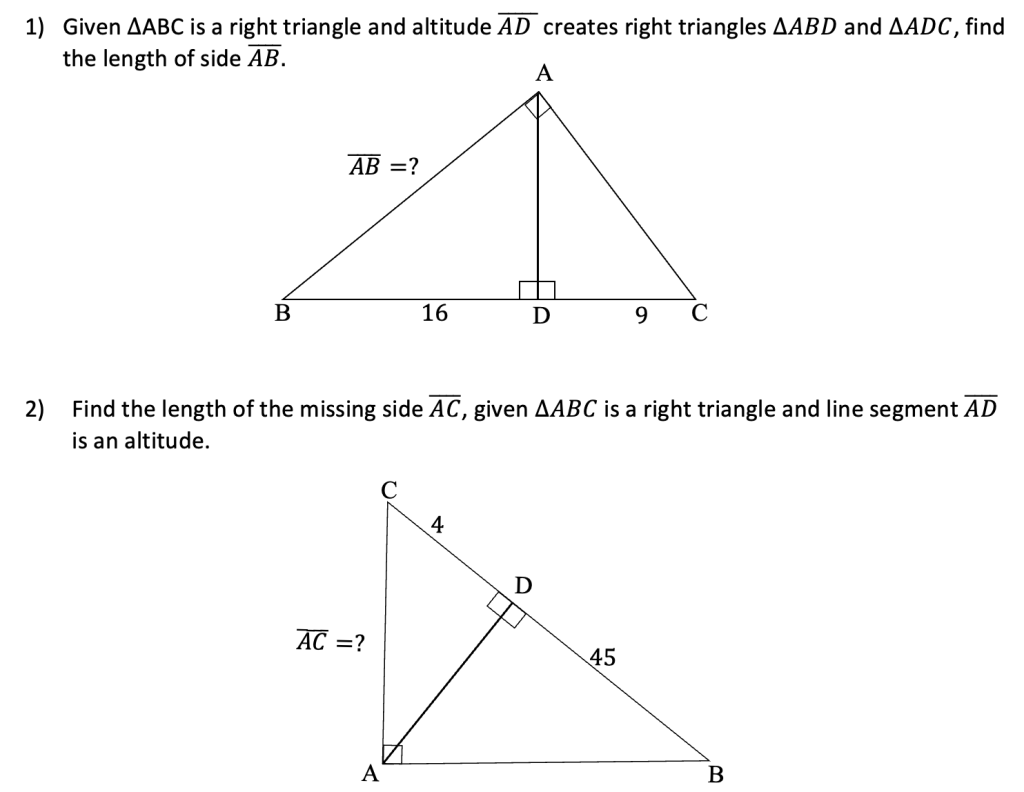
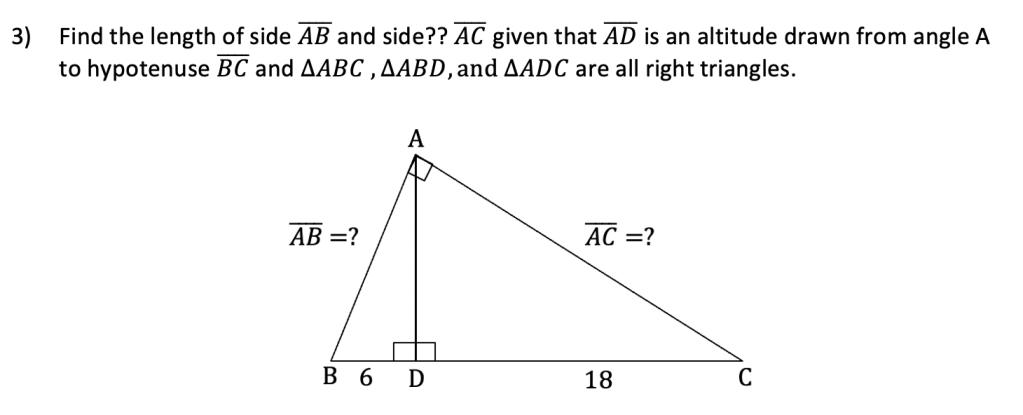
Practice Questions:
Solutions:
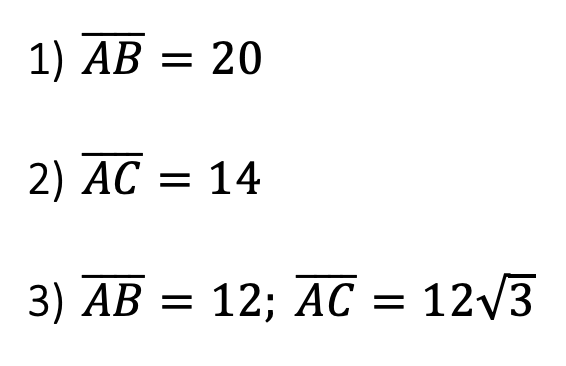
Still got questions? No problem! Don’t hesitate to comment with any questions below. Thanks for stopping by and happy calculating!
Facebook ~ Twitter ~ TikTok ~ Youtube
Check out more posts on Similar Triangles here!





![Erratum for “An inverse theorem for the Gowers U^s+1[N]-norm”](https://azmath.info/wp-content/uploads/2024/07/2211-erratum-for-an-inverse-theorem-for-the-gowers-us1n-norm-150x150.jpg)

