Many math resources attempt to share the difference between teaching FOR problem solving and teaching THROUGH problem solving. Cathy Seeley refers to teaching THROUGH problem solving as “Upside-Down Teaching” which is the opposite of a “gradual release of responsibility” model:
And instead calls for us to flip how our students learn to a more active model:
So, instead of starting a unit on Geometry with naming shapes or developing definitions together, we decided to start with a little problem:
Create as many polygons as possible using exactly 2 pattern block pieces. Sort your polygons by how many sides they have.
As students started placing pattern block pieces together, all kinds of questions started emerging (questions we took note of to bring to the whole group in a few minutes):
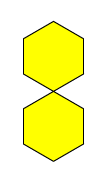
By the end of a period, students had worked through the definitions of what a polygon is (and isn’t), the difference between concave and convex polygons, defined the term “regular polygon” (which was not what they had been calling “regular” before), and were able to name and create triangles, quadrilaterals, pentagons, hexagons, heptagons, octagons, nonagons, decagons and undecagons. Recognizing a variety of possible ways a shape can look was very helpful for our students who might have experienced shapes more traditionally in the past.
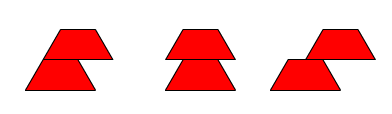
One group compiled their polygons together (with one minor error):
Instead of starting with experiences where students accumulate knowledge (writing out definitions, taking a note, direct instruction), an upside down approach aims to start with students’ ideas. This way we would know which conversations to have with our students, and so our students are actively engaged in the process of learning.
I want to leave you with a few reflective questions:
- Why might it benefit students to start with a problem instead of starting with the teachers’ ideas?
- Why might it benefit teachers to listen to students’ thinking before instruction has occurred?
- What does it mean to effectively monitor students as they are thinking / working? (See This POST for examples)
- Can all mathematics topics begin with tasks that help our students make connections between what they already know, and what they are learning? Can you think of a topic that can not be experienced this way?
- The final stage in the You-We-I model is where the teacher helps make specific learning explicit for their students. How do you find time to consolidate a task like this? How do you know what to share? (See This POST for an example)
- How might this form of teaching relate to how we view assessment? (See This POST)
- How might this form of teaching relate to how we view differentiated instruction? (See This POST)
- How do you find problems that ask students to actively think before any instruction has occurred? (See This POST for examples)
I’d love to continue the conversation. Feel free to write a response, or send me a message on Twitter ( @markchubb3 ).
