Ask any teacher anywhere what some of the most pressing challenges are that they face as a teacher and likely you will hear examples of how difficult it can be to meet the various needs within a classroom. When conversations on the topic arise, there are often discussions from one of two extremes:

One one side you might hear about reasons why a teacher might believe that it is best to make sure that every student be expected to learn the same things. These beliefs often lead to practices where everyone receives the same instruction, followed by individual assistance for students who were not able to follow classroom instructions. Equity here is believed to be related to equal outcomes.
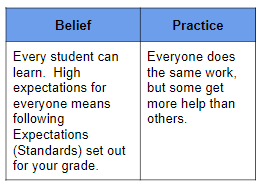
On the other hand, some teachers might believe that it isn’t possible to expect every student to learn the same things at the same time. Their beliefs lead them to focus more attention on determining readiness and offering different groups of students different learning opportunities. Equity here is viewed as meeting each child’s unique need.
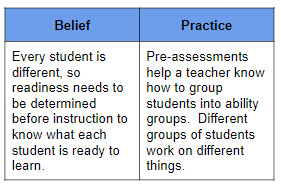
While I understand each of these points of view, part of the issue between these two views is the overall view of what mathematics is. If mathematics is seen as a set of linearly learned skills, where each skill is boiled down to a list of subskills, each learned in a specific sequence, it is difficult to imagine anything else. However, when mathematics is seen through the lens of rich connections, we might start to see students’ development of these connections as what can drive our decisions.
One way to think of a person’s understanding of mathematics is that it exists along a continuum. At one end is a rich set of connections. At the other end of the continuum, ideas are isolated or viewed as disconnected bits of information. A sound understanding of mathematics is one that sees the connections within mathematics and between mathematics and the world.
TIPS4RM: Developing Mathematical Literacy, 2005
The two views mentioned above do not account for a view of mathematics where connections between concepts is a focus. Instead of seeing the issue as simply whether or not we want a classroom of students to be doing the same things or if we should be providing some students with different things, we should also consider what is actually being learned by the students. Below you can see a matrix showing four different examples of how we could tackle the same concept in a classroom:
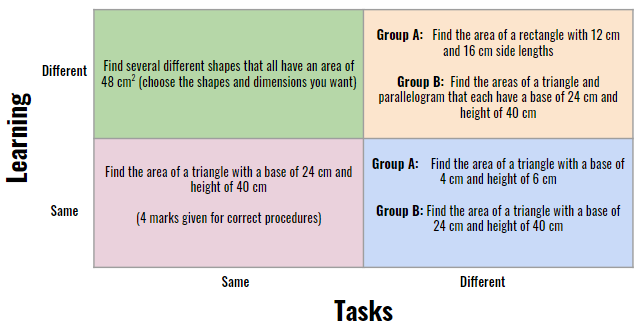
Same / Different Learning? Same / Different Tasks?
Same Tasks, Same Learning: The teacher offers everyone the same task, expects everyone to be able to follow the same procedures and might offer explicit help to specific students that aren’t following accordingly.
Different Tasks, Same Learning: While everyone is learning the same thing, the teacher offers some groups easier work and other groups more advanced work based on readiness.
Different Tasks, Different Learning: Based on diagnostic assessments, students are placed into groups based on what they need to continue learning. Some groups might be learning different materials within the same class.
Same Tasks, Different Learning: Every student is provided the same task, but there is variance in how and what is being learned.
For the readers here, I encourage you to think about which of the above models might you have experienced as a student, and which you might think would be best for your students.
Taking an Equity Stance
So, what does any of this have to do with equity? In my experience, a lot! Taking an equity stance means that we both believe that every student can achieve, and understand that every student might need different things from us. Keeping equity in mind requires us to analyze who has access to rich mathematical experiences and whose experiences are narrowed or reduced to lower-level skills (Access), whose ideas contribute to the group’s development of mathematical understanding and whose are not heard (Agency and Authority), who identifies with mathematics and who does not (Identity)… Without considering our beliefs and practices, we will never be able to notice which students are being underserviced, which structures promote some groups over others, or see which practices lead to the “Matthew Effect“.
How do we aim for Equity?
When thinking about how we aim for equity in mathematics, there seems to be 2 key tenets that help point us in the right direction:
- Expand WHO is considered a math student
- Expand WHAT is accepted as mathematics
The question is not whether all students can succeed in mathematics but whether the adults organizing mathematics learning opportunities can alter traditional beliefs and practices to promote success for all.
Principles to Action – NCTM (p.61)
WHO is considered a math person?
Teachers who come to recognize that some students identify with mathematics (and others do not) aim to promote tasks that allow more students to engage in mathematical reasoning via problems/tasks that are easily accessible (low-floor, high-ceiling tasks). If our students are going to see themselves as budding mathematicians, then we need to allow more opportunities for students to share their emerging ideas first!
Dr. Christine Suurtamm does a great job of articulating what this could look like in practice:
Students need to see themselves in the work they are doing. This includes knowing that mathematics is not created for and used by only some people (race/gender…), nor is it only useful for potential futures of some of our students, but is actually used by all of us RIGHT NOW. If we want to make sure our students see themselves as mathematicians, OUR STUDENTS need to be doing more of the thinking, they need to be part of the process of learning, not simply showing that they have mastered procedures.
Reflecting on WHO believes they are a math person might help us reflect on what messages our students have received over the years. If you haven’t already read about the “Matthew Effect“, I recommend that this might be a great place to help you reflect.
WHAT Counts as “Mathematics”?
To some, mathematics is a very narrow subject. Calculating (adding, subtracting, multiplying, dividing), converting (equivalent fractions), and carrying out other procedures accurately by using the requisite steps… Procedures dominate some textbooks and online practice sites and for some, this narrow vision of mathematics is where some students begin to struggle. However, if we are aiming for equity then we need to allow more opportunities for our students to show us what ARE good at.
One way to expand what counts as mathematics is for us to reflect on how much time we spend on each strand of mathematics (Patterning, Number Sense, Geometry, Measurement, Data Management). Analyzing how much time we spend on each of these strands, and specifically when in the year we might teach these concepts might help us reflect on what messages our students hear when they consider what counts as mathematics. For example, schools in my area typically start with several weeks of patterning because it can be experienced physically (manipulatives) and visually (visual patterns, graphing…), followed by several weeks of Geometry. These moves were strategic, because it allows our students more opportunities to talk, more opportunities to solve problems, more opportunities for our students to use visual/ spatial reasoning and more students to start their year with successes!
Expanding what mathematics means is much more than strands or concepts though. A focus on concrete and visual representations (not solely abstract symbolic representations) can be a path to expand what counts as mathematics. Allowing students to show their strategies, and accepting student strategies as part of the learning process means that preformal representations and strategies can be compared and learned from.
Spatial puzzles and games allow students to think mathematically in ways that differ from typical assignments. A story I often tell is of this young student who had never liked mathematics, and often struggled with mathematics. Here you can see her attempting to solve a difficult puzzle that one of her classmates created. Every child deserves to experience what this student experienced – productive struggle and success. Take a look:
Considerations
If we are aiming for equity in our own personal practices, we need to be aware of our own biases, our own patterns. This isn’t easy! It might mean noticing how we talk about race or gender or socio-economic groups and what our expectations are for each. It might mean reflecting on words we use to discuss students who might currently be struggling to learn mathematics or who are identified learners and what our expectations are of these students. Again, learning more about the Matthew Effect is where I would recommend you start. Planning with providing greater access for students to learn mathematics (same tasks/different learning – spatializing mathematics) is likely a first concrete step we can take.
I want to leave you with a few reflective questions:
- How do you see the Same/Different Learning – Same/Different Tasks chart relating to equity? Which quadrant would you like provide for your students to be engaged with more frequently? What barriers are standing in the way?
- We need to be aware that when schools group students by ability or assign different tasks to different students, those that are relegated to lower groups/tasks often receive lower level of cognitive demand tasks, which often puts them at a further disadvantage than their peers. How do you combat these inequities in your classroom?
- Providing students with rich tasks and access to rich problems isn’t enough. We also need to be noticing our students’ thinking so we know how to respond to our students individually and as a group. This isn’t easy! How do you pay attention to their thinking? What structures do you have in place to listen to students and respond accordingly?
- How do you monitor your students’ interests and thoughts about mathematics in general, or about specific concepts?
- How are you aiming to minimize the Matthew Effect and reduce inequities in your room?
As always, I’d love to hear your thoughts. Leave a reply here on Twitter (@MarkChubb3)





![Erratum for “An inverse theorem for the Gowers U^s+1[N]-norm”](https://azmath.info/wp-content/uploads/2024/07/2211-erratum-for-an-inverse-theorem-for-the-gowers-us1n-norm-150x150.jpg)

