Whose problems? Whose game? Whose puzzle? – Thinking Mathematically

TRU Math (Teaching for Robust Understanding) a few years ago shared their thoughts about what makes for a “Powerful Classroom”. Here are their 5 dimensions:

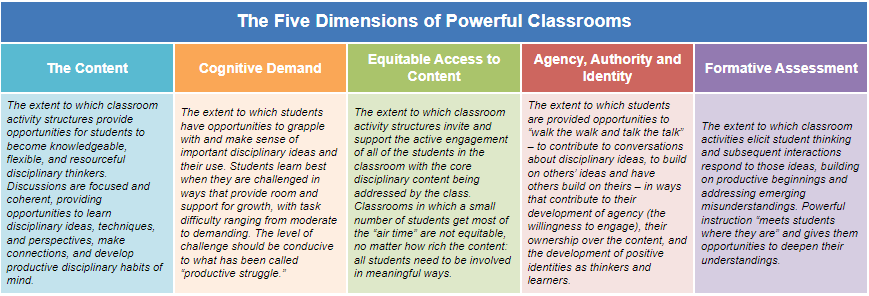
Looking through the dimensions here, it is obvious that some of these dimensions are discussed in detail in professional development sessions and in teacher resources. However, the dimension of Agency, Authority and Identity is often overlooked – maybe because it is much more complicated to discuss. Take a look at what this includes:

This dimension helps us as teachers consider our students’ perspectives. How are they experiencing each day? We should be reflecting on:

Who has a voice? Who doesn’t?
How are ideas shared between and among students?
Who feels like they have contributed? Who doesn’t?
Who is actively contributing? Who isn’t?

Reflecting on our students’ experiences makes us better teachers! So, I’ve been wondering:

Who created today’s problem / game / puzzle?

For most students, math class follows the same pattern:

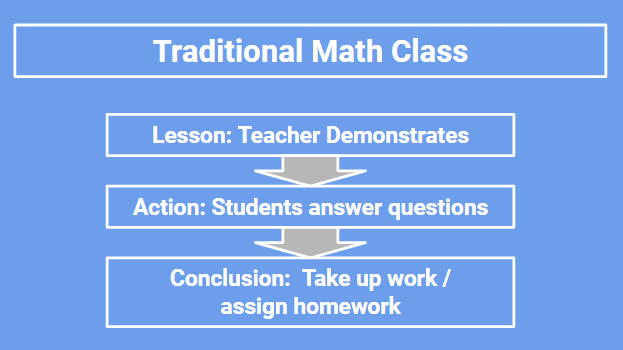
This pattern of a lesson leaves many students disinterested, because they are not actively involved in the learning – which might lead to typical comments like, “When will we ever need this?”. This lesson format is TEACHER centered because it centers the teachers’ ideas (the teacher provides the problem, the teacher helps students, the teacher tells you if you are correct). In this example, students’ mathematical identities are not fostered. There is NO agency afforded to students. Authority solely belongs to the teachers. But there are ways to make identity / agency / authority a focus!

STUDENT driven ideas

Today as a quick warm-up, I had students solve a little pentomino puzzle. After they finished, I asked students to create their own puzzles that others will solve. Here is one of the student created puzzles:

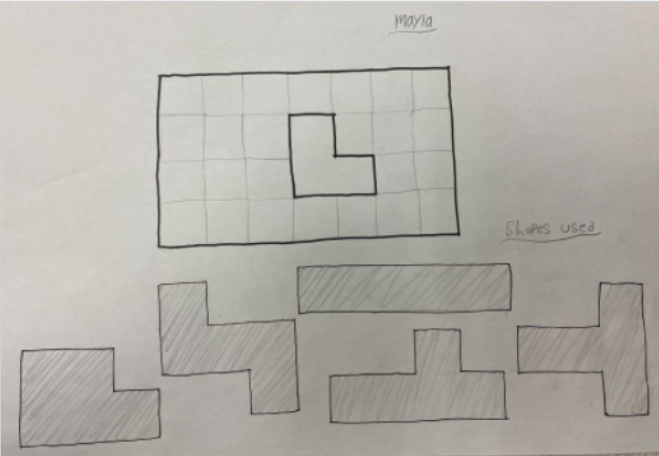
Here you can see a simple puzzle. The pieces are shown that you must use, and the board is included (with a hole in the middle). Now, as a class, we have a bank of puzzles we can attempt any day (as a warm-up or if work is finished).

You can read about WHY we would do puzzles like this in math class along with some examples (Spatial Reasoning).

What’s more important here is for us to reflect on how we are involving our own students in the creation of problems, games and puzzles in our class. This is a low-risk way to allow everyone in class do more than just participate, they are taking ownership in their learning, and building a community of learners that value learning WITH and FROM each other!

How to involve our students?

The example above shows us a simple way to engage our students, to expand what we consider mathematics and help our students form positive mathematical identities. However, there are lots of ways to do this:

Play a math game for a day or 2, then ask students to alter one or a few of the rules.
Have students submit questions you might want to consider for an assessment opportunity.
Have students look through a bank or questions / problems and ask which one(s) would be the most important ones to do.
Give students a sheet of many questions. Ask them to only do the 3 easiest, and the 3 hardest (then lead a discussion about what makes those ones the hardest).
Lead 3-part math lessons where students start by noticing / wondering.
Have students design their own SolveMe mobile puzzles, visual patterns, Which One Doesn’t Belong…

Questions to Reflect on:

Who is not contributing in your class, or doesn’t feel like they are a “math student”? Whose mathematical identities would you like to foster? How might something simple like this make a world of difference for those children?
Does it make a difference WHO develops the thinking?
Fostering student identities, paying attention to who has authority in your class and allowing students to take ownership is essential to build mathematicians. The feeling of belonging in this space is crucial. How are you paying attention to this? (See Matthew Effect)
How might these ideas help you meet the varied needs within a mixed ability classroom?
If you do have your students create their own puzzles, will you first offer a simplified version so your students get familiar with the pieces, or will you dive into having them make their own first?
Would you prefer all of your students doing the same puzzle / game / problem, or have many puzzles / games / problems to choose from? How might this change class conversations afterward?
As the teacher, what will you be doing when students are playing? How might listening to student thinking help you learn more about your students? (See: Noticing and Wondering: A powerful tool for assessment)

I’d love to continue the conversation about students’ identities in mathematics. Leave a comment here or on Twitter @MarkChubb3